1 5 Study Guide And Intervention Angle Relationships
Onlines
Apr 06, 2025 · 6 min read
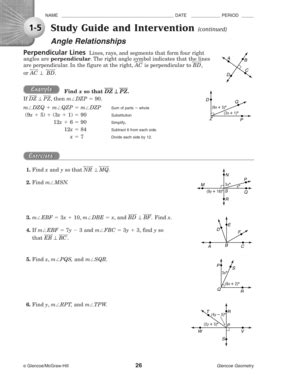
Table of Contents
1-5 Study Guide and Intervention: Angle Relationships
This comprehensive guide delves into the fascinating world of angle relationships, specifically focusing on the key concepts covered in a typical 1-5th-grade mathematics curriculum. We'll explore different types of angles, their properties, and how they interact with each other. Understanding angle relationships is crucial for building a strong foundation in geometry and higher-level mathematics. This guide provides explanations, examples, and practice problems to solidify your understanding. Let's begin!
Understanding Basic Angle Types
Before diving into the relationships between angles, let's refresh our knowledge of fundamental angle types. These are the building blocks upon which more complex concepts are built.
1. Acute Angles:
An acute angle measures less than 90 degrees. Think of it as a small, sharp angle. Imagine a slice of pizza that's quite thin – that's an acute angle!
Example: An angle measuring 30 degrees is an acute angle.
2. Right Angles:
A right angle measures exactly 90 degrees. It's often represented by a small square in the corner of the angle. Think of the corner of a perfectly square piece of paper.
Example: The corner of a book or a square tile forms a right angle.
3. Obtuse Angles:
An obtuse angle measures more than 90 degrees but less than 180 degrees. It's a wider angle than a right angle. Imagine opening a door more than halfway – that's an obtuse angle!
Example: An angle measuring 120 degrees is an obtuse angle.
4. Straight Angles:
A straight angle measures exactly 180 degrees. It forms a straight line. Think of a perfectly flat, extended arm.
Example: A straight line on a ruler represents a straight angle.
5. Reflex Angles:
A reflex angle measures more than 180 degrees but less than 360 degrees. It's a large angle that extends beyond a straight line. Think of more than half a circle.
Example: An angle measuring 270 degrees is a reflex angle.
Exploring Angle Relationships
Now that we've covered the basic angle types, let's explore how angles interact with each other. Understanding these relationships is vital for solving geometric problems.
1. Complementary Angles:
Two angles are complementary if their measures add up to 90 degrees. They often appear together to form a right angle.
Example: A 30-degree angle and a 60-degree angle are complementary because 30 + 60 = 90.
2. Supplementary Angles:
Two angles are supplementary if their measures add up to 180 degrees. They often appear together to form a straight line.
Example: A 110-degree angle and a 70-degree angle are supplementary because 110 + 70 = 180.
3. Vertical Angles:
Vertical angles are formed when two lines intersect. They are the angles opposite each other. Vertical angles are always congruent (equal in measure).
Example: When two lines cross, the angles directly opposite each other are vertical angles and are equal in measure. If one vertical angle measures 45 degrees, the other vertical angle also measures 45 degrees.
4. Adjacent Angles:
Adjacent angles are angles that share a common vertex (corner point) and a common side. They are next to each other.
Example: Two angles that are side-by-side forming part of a larger angle are adjacent.
5. Linear Pair:
A linear pair is a special case of adjacent angles. A linear pair consists of two adjacent angles that are supplementary (add up to 180 degrees). They form a straight line.
Example: Two adjacent angles forming a straight line always constitute a linear pair. If one angle in a linear pair measures 135 degrees, the other angle measures 45 degrees (180 - 135 = 45).
Problem Solving with Angle Relationships
Let's put our knowledge to the test with some practice problems. These examples will help you solidify your understanding of angle relationships.
Problem 1: Two angles are complementary. One angle measures 25 degrees. What is the measure of the other angle?
Solution: Since the angles are complementary, their sum is 90 degrees. Therefore, the other angle measures 90 - 25 = 65 degrees.
Problem 2: Two angles are supplementary. One angle measures 115 degrees. What is the measure of the other angle?
Solution: Since the angles are supplementary, their sum is 180 degrees. Therefore, the other angle measures 180 - 115 = 65 degrees.
Problem 3: Two lines intersect, forming four angles. One angle measures 70 degrees. What are the measures of the other three angles?
Solution: Vertical angles are equal, so the angle opposite the 70-degree angle also measures 70 degrees. The other two angles are supplementary to the 70-degree angle, so they each measure 180 - 70 = 110 degrees.
Problem 4: Find the value of x if two adjacent angles are represented by (3x + 10)° and (2x - 5)° and they form a right angle.
Solution: Since they form a right angle, their sum is 90 degrees: (3x + 10) + (2x - 5) = 90. Solving for x, we get 5x + 5 = 90, 5x = 85, x = 17. Therefore, the angles measure (317 + 10) = 61° and (217 - 5) = 29°. 61° + 29° = 90°.
Problem 5: Two angles form a linear pair. One angle is three times the measure of the other. Find the measure of each angle.
Solution: Let one angle be x. The other angle is 3x. Since they form a linear pair, their sum is 180 degrees: x + 3x = 180. This simplifies to 4x = 180, so x = 45. Therefore, one angle measures 45 degrees, and the other measures 3 * 45 = 135 degrees.
Advanced Concepts (for higher grades within the 1-5 range)
As students progress through grades 1-5, the complexity of angle relationship problems increases. Here are some advanced concepts that might be introduced in later grades:
-
Triangles and Angle Sums: The sum of the angles in any triangle is always 180 degrees. This concept can be used to solve for unknown angles within triangles.
-
Polygons and Angle Sums: Understanding the relationship between the number of sides of a polygon and the sum of its interior angles becomes relevant in higher grades. Formulas are introduced to calculate these sums.
-
Isosceles and Equilateral Triangles: These special triangles have properties related to their angles. Isosceles triangles have two equal angles, and equilateral triangles have three equal angles (each measuring 60 degrees).
-
Applying Angle Relationships in Real-World Scenarios: Problems involving real-world applications, such as calculating angles in architectural designs or measuring angles of objects in the environment, can help students see the practical applications of angle relationships.
Conclusion
Understanding angle relationships is fundamental to success in geometry and beyond. Mastering the concepts of acute, obtuse, right, straight, and reflex angles, along with complementary, supplementary, vertical, adjacent, and linear pairs, provides a strong foundation for more advanced mathematical concepts. Through consistent practice and application, students can develop a deep understanding of these relationships and confidently tackle challenging problems. Remember to break down complex problems into smaller, manageable steps and always visualize the angles to gain a better grasp of the concepts. Keep practicing, and you’ll become a geometry expert in no time!
Latest Posts
Latest Posts
-
Introduction To Energy Worksheet Answer Key
Apr 09, 2025
-
Judith Butler Psychic Life Of Power
Apr 09, 2025
-
Themes For A Long Walk To Water
Apr 09, 2025
-
We Can Define Sport In General Terms As
Apr 09, 2025
-
Roberto Y Yo Levantarse A Las 7
Apr 09, 2025
Related Post
Thank you for visiting our website which covers about 1 5 Study Guide And Intervention Angle Relationships . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.