5.5 Determine Absolute Extrema From Candidates
Onlines
Mar 28, 2025 · 6 min read
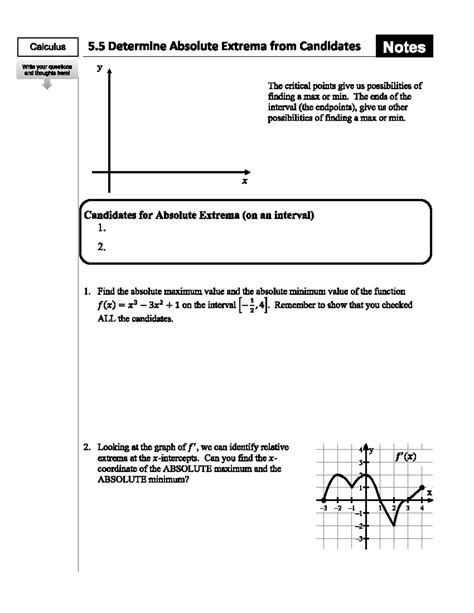
Table of Contents
5.5 Determining Absolute Extrema from Candidates: A Comprehensive Guide
Finding absolute extrema—the absolute maximum and minimum values of a function on a given interval—is a crucial concept in calculus with wide-ranging applications in optimization problems across various fields. While understanding derivatives and critical points is essential, the final step often involves evaluating the function at specific candidate points to definitively determine the absolute extrema. This guide delves into the process of determining absolute extrema from these candidates, providing a step-by-step approach with illustrative examples.
Understanding the Candidates
Before we dive into the process, let's clarify what constitutes a "candidate" for an absolute extremum. Candidates are points within the domain of the function where an extremum might occur. These points typically include:
-
Critical Points: These are points where the derivative of the function is either zero or undefined. Critical points are potential locations for local extrema (maxima or minima within a smaller interval). However, not all critical points are absolute extrema.
-
Endpoints of the Interval: If the problem specifies a closed interval [a, b], the endpoints a and b must be considered as candidates. Absolute extrema can often occur at the boundaries of the interval.
The Systematic Approach: A Step-by-Step Guide
Determining absolute extrema from candidates involves a straightforward, systematic approach. Follow these steps to ensure accuracy and avoid common pitfalls:
Step 1: Find the Critical Points
This involves calculating the first derivative of the function, f'(x), and solving for x when f'(x) = 0 or f'(x) is undefined. Remember to check the domain of the function to identify any points where the derivative might be undefined (e.g., division by zero, square roots of negative numbers).
Step 2: Identify the Endpoints (if applicable)
If the problem involves a closed interval [a, b], note the endpoints a and b as candidates. These are crucial as absolute extrema can occur at the boundaries.
Step 3: Evaluate the Function at Each Candidate
Substitute each candidate point (critical points and endpoints) into the original function, f(x), to calculate the corresponding y-values. This step generates a list of potential y-values for the absolute extrema.
Step 4: Compare the Function Values
Compare all the y-values obtained in Step 3. The largest y-value represents the absolute maximum, and the smallest y-value represents the absolute minimum of the function on the given interval.
Step 5: State the Absolute Extrema
Clearly state the absolute maximum and minimum values, along with the x-values at which they occur. Remember to provide the x and y coordinates (x, y) for each extremum.
Illustrative Examples: From Simple to Complex
Let's solidify this understanding with several examples, starting with simpler cases and progressing to more complex scenarios.
Example 1: A Simple Quadratic Function
Find the absolute extrema of the function f(x) = x² - 4x + 3 on the interval [0, 3].
-
Critical Points: f'(x) = 2x - 4. Setting f'(x) = 0 gives 2x - 4 = 0, so x = 2.
-
Endpoints: The endpoints are x = 0 and x = 3.
-
Evaluation:
- f(0) = 3
- f(2) = -1
- f(3) = 0
-
Comparison: The largest y-value is 3 (at x = 0), and the smallest is -1 (at x = 2).
-
Result: The absolute maximum is (0, 3), and the absolute minimum is (2, -1).
Example 2: A Function with Undefined Derivative
Find the absolute extrema of f(x) = √x on the interval [0, 4].
-
Critical Points: f'(x) = 1/(2√x). The derivative is undefined at x = 0.
-
Endpoints: The endpoints are x = 0 and x = 4.
-
Evaluation:
- f(0) = 0
- f(4) = 2
-
Comparison: The largest y-value is 2 (at x = 4), and the smallest is 0 (at x = 0).
-
Result: The absolute maximum is (4, 2), and the absolute minimum is (0, 0).
Example 3: A Function with Multiple Critical Points
Find the absolute extrema of f(x) = x³ - 3x² + 2 on the interval [-1, 3].
-
Critical Points: f'(x) = 3x² - 6x = 3x(x - 2). Setting f'(x) = 0 gives x = 0 and x = 2.
-
Endpoints: The endpoints are x = -1 and x = 3.
-
Evaluation:
- f(-1) = -2
- f(0) = 2
- f(2) = -2
- f(3) = 2
-
Comparison: The largest y-value is 2 (at x = 0 and x = 3), and the smallest is -2 (at x = -1 and x = 2).
-
Result: The absolute maximum is (0, 2) and (3, 2), and the absolute minimum is (-1, -2) and (2, -2). Notice that a function can have multiple points with the same absolute maximum or minimum value.
Example 4: A Function on an Open Interval
Finding absolute extrema on an open interval (a, b) requires careful consideration. Since there are no endpoints, absolute extrema might not exist. The function might approach infinity or negative infinity as x approaches a or b.
Consider f(x) = 1/x on the interval (0, 1). The derivative is f'(x) = -1/x², which is always negative. There are no critical points. As x approaches 0 from the right, f(x) approaches infinity, and as x approaches 1, f(x) approaches 1. Therefore, there is no absolute maximum on this open interval. The infimum is 1, but it is not an absolute minimum, as the function never actually reaches the value 1.
Handling More Complex Scenarios
While the basic steps remain the same, more complex scenarios might involve:
-
Functions with multiple variables: Techniques like Lagrange multipliers or finding critical points using partial derivatives become necessary.
-
Functions defined piecewise: You'll need to analyze each piece of the function separately, considering the endpoints of each piece as potential candidates.
-
Functions with discontinuities: Discontinuities can introduce additional candidate points where the function's value may abruptly change.
Applications of Finding Absolute Extrema
The ability to find absolute extrema is vital in many fields, including:
-
Optimization problems: Finding the maximum profit, minimum cost, or optimal design often involves identifying the absolute extrema of a relevant function.
-
Engineering: Determining maximum stress on a structure or minimizing material usage.
-
Economics: Maximizing revenue or minimizing production costs.
-
Physics: Determining the maximum height of a projectile or the minimum energy state of a system.
Conclusion
Determining absolute extrema from candidate points is a fundamental skill in calculus. By following the systematic approach outlined in this guide—finding critical points, identifying endpoints, evaluating the function at candidate points, comparing function values, and stating the results clearly—you can confidently solve a wide range of optimization problems. Remember that careful consideration of the function's behavior and the interval of interest are crucial for accurate and complete solutions. The examples provided illustrate the process for various scenarios, from simple to more complex cases, providing a strong foundation for tackling diverse applications.
Latest Posts
Latest Posts
-
Select All Of The Events That Happen In Prophase
Mar 31, 2025
-
Air Pollution Is Accurately Described By Which Statement
Mar 31, 2025
-
Family Counseling Approach Research Paper Assignment
Mar 31, 2025
-
How To Check Your Glo Number
Mar 31, 2025
-
What Are 4 Purposes Of Government
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about 5.5 Determine Absolute Extrema From Candidates . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
