7.8 Exponential Models With Differential Equations
Onlines
Apr 07, 2025 · 5 min read
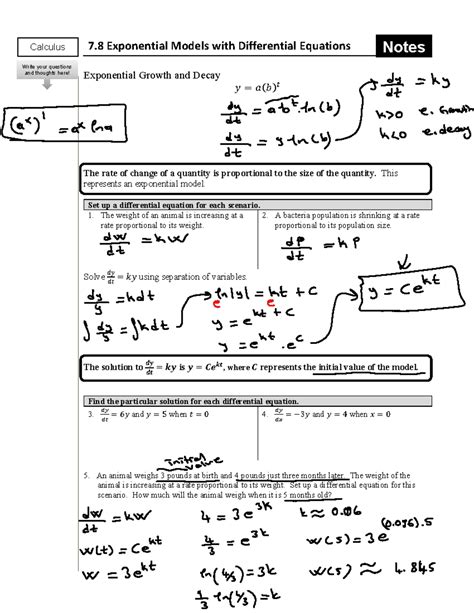
Table of Contents
7.8 Exponential Models with Differential Equations: A Comprehensive Guide
Exponential models, characterized by their rapid, sustained growth or decay, are ubiquitous in various scientific and engineering disciplines. Understanding these models is crucial for analyzing phenomena ranging from population dynamics and radioactive decay to the spread of infectious diseases and compound interest. Differential equations provide a powerful mathematical framework for describing the continuous change inherent in exponential processes. This article delves into the intricacies of 7.8 exponential models within the context of differential equations, providing a comprehensive overview for students and researchers alike.
Understanding Exponential Growth and Decay
Before delving into the intricacies of differential equations, let's establish a firm understanding of the fundamental principles of exponential growth and decay.
Exponential Growth
Exponential growth describes a scenario where a quantity increases at a rate proportional to its current value. The larger the quantity, the faster it grows. This is mathematically represented by the equation:
P(t) = P₀e^(kt)
Where:
- P(t) is the quantity at time t.
- P₀ is the initial quantity at time t = 0.
- k is the growth rate constant (k > 0).
- e is the base of the natural logarithm (approximately 2.71828).
The constant k dictates the speed of growth. A larger k signifies faster growth.
Exponential Decay
Exponential decay mirrors exponential growth, but instead of increasing, the quantity decreases at a rate proportional to its current value. The mathematical representation is:
P(t) = P₀e^(-kt)
Where:
- P(t) is the quantity at time t.
- P₀ is the initial quantity at time t = 0.
- k is the decay rate constant (k > 0).
- e is the base of the natural logarithm.
Here, a larger k indicates faster decay.
Differential Equations and Exponential Models
Differential equations elegantly describe the rate of change of a quantity. For exponential growth and decay, this rate of change is directly proportional to the quantity itself. This leads to the following differential equations:
Differential Equation for Exponential Growth:
dP/dt = kP
This equation states that the rate of change of P with respect to time (dP/dt) is equal to k times P.
Differential Equation for Exponential Decay:
dP/dt = -kP
The negative sign signifies the decrease in quantity over time.
Solving the Differential Equations
Solving these differential equations yields the familiar exponential growth and decay formulas. The process typically involves separation of variables and integration.
Solving the Exponential Growth Equation:
-
Separate Variables: (dP/P) = k dt
-
Integrate both sides: ∫(dP/P) = ∫k dt => ln|P| = kt + C₁ (where C₁ is the constant of integration)
-
Solve for P: P = e^(kt + C₁) = e^(kt) * e^(C₁) = P₀e^(kt) (where P₀ = e^(C₁) is the initial condition)
Solving the Exponential Decay Equation:
The process is analogous to the growth equation, except for the negative sign:
-
Separate Variables: (dP/P) = -k dt
-
Integrate both sides: ∫(dP/P) = ∫-k dt => ln|P| = -kt + C₁
-
Solve for P: P = e^(-kt + C₁) = e^(-kt) * e^(C₁) = P₀e^(-kt)
Applications of 7.8 Exponential Models
The versatility of exponential models makes them applicable across diverse fields:
1. Population Growth:
Modeling population growth, especially in the early stages before resource limitations become significant, often employs exponential growth models. The growth rate k represents the birth rate minus the death rate.
2. Radioactive Decay:
Radioactive decay follows an exponential decay model. The decay constant k is related to the half-life of the radioactive substance. This has crucial applications in carbon dating and nuclear medicine.
3. Compound Interest:
The growth of money in a savings account with compound interest is an example of exponential growth. The interest earned is added to the principal, leading to exponential growth of the total amount.
4. Spread of Infectious Diseases:
In the early stages of an epidemic, before interventions or herd immunity take effect, the spread of an infectious disease can be modeled using exponential growth.
5. Cooling and Heating:
Newton's Law of Cooling states that the rate of change of temperature of an object is proportional to the difference between its temperature and the ambient temperature. This leads to an exponential model describing the cooling or heating process.
Limitations of Exponential Models
While incredibly useful, exponential models have limitations:
-
Unrealistic Growth: Exponential growth models assume unlimited resources and space, which is rarely true in real-world scenarios. Population growth, for instance, eventually levels off due to resource constraints.
-
Constant Growth Rate: The constant growth or decay rate k might not be accurate for all time periods. Factors like changes in environmental conditions or government policies can significantly affect the growth rate.
-
Simplification: Exponential models simplify complex phenomena by assuming a constant rate of change. In reality, many processes are more nuanced and might require more sophisticated models.
Advanced Exponential Models: Incorporating Other Factors
To address some of the limitations of simple exponential models, more complex models are employed that incorporate additional factors:
-
Logistic Growth: The logistic growth model incorporates carrying capacity, representing the maximum population size the environment can sustain. This model shows initial exponential growth followed by a gradual leveling off.
-
Gompertz Growth: The Gompertz growth model is another alternative that incorporates a slowing growth rate as the population approaches its maximum.
-
Modified Exponential Models: Various modifications can be introduced to account for variations in the growth or decay rate over time. This could involve time-dependent parameters or incorporating periodic fluctuations.
Conclusion
7.8 exponential models, described through differential equations, provide a powerful tool for analyzing a wide range of phenomena exhibiting exponential growth or decay. Understanding the underlying principles, solving the associated differential equations, and recognizing the limitations of these models are critical skills for anyone working in fields involving continuous change. While simple exponential models offer a starting point, more sophisticated models are often necessary to capture the complexities of real-world processes. Continued exploration of these models and their variations will remain vital for accurate predictions and informed decision-making across diverse scientific and engineering disciplines. The ability to adapt and modify these models to incorporate additional factors will enhance their predictive capabilities and allow for a more nuanced understanding of dynamic systems.
Latest Posts
Latest Posts
-
Identify The True And False Statements About Race
Apr 08, 2025
-
What Does Fences Symbolism In Fences
Apr 08, 2025
-
Attitudes And Behaviors Come From Our Blank System
Apr 08, 2025
-
Ap Euro Unit 4 Progress Check Mcq
Apr 08, 2025
-
Vertical Stretching And Compressing Functions Homework Answers
Apr 08, 2025
Related Post
Thank you for visiting our website which covers about 7.8 Exponential Models With Differential Equations . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.