A Block Initially At Rest Is Given A Quick Push
Onlines
Apr 01, 2025 · 7 min read
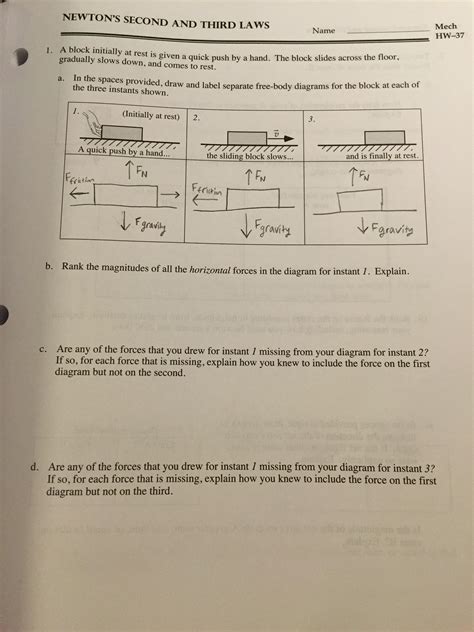
Table of Contents
A Block Initially at Rest: Exploring the Physics of a Quick Push
A seemingly simple scenario – a block initially at rest receiving a quick push – actually unveils a rich tapestry of physics principles. This seemingly mundane event allows us to explore fundamental concepts like Newton's Laws of Motion, impulse, momentum, friction, and even delve into more advanced topics like energy transfer and collisions. This article will dissect this seemingly simple act, providing a comprehensive understanding of the forces at play and the resulting motion.
Newton's Laws: The Foundation of Motion
The cornerstone of understanding the motion of the block lies in Newton's three laws of motion:
Newton's First Law: Inertia
Initially, the block is at rest. This state of rest is maintained due to inertia, the tendency of an object to resist changes in its state of motion. The block remains at rest unless acted upon by an external, unbalanced force. This external force is provided by the "quick push."
Newton's Second Law: F=ma
The "quick push" exerts a force (F) on the block. Newton's second law states that the net force acting on an object is equal to the product of its mass (m) and its acceleration (a): F = ma. This equation is crucial in determining the block's acceleration. The greater the force of the push, the greater the acceleration; the greater the mass of the block, the smaller the acceleration for the same force.
Newton's Third Law: Action-Reaction
For every action, there's an equal and opposite reaction. When you push the block, you exert a force on it. Simultaneously, the block exerts an equal and opposite force on your hand. This is why you might feel a slight resistance when pushing the object.
The Role of Impulse and Momentum
The "quick push" isn't a sustained force; it's applied over a short duration. This introduces the concepts of impulse and momentum:
Impulse: The Change in Momentum
Impulse (J) is the change in momentum (Δp) of an object. It's calculated as the product of the average force (F<sub>avg</sub>) applied and the time interval (Δt) over which the force acts: J = F<sub>avg</sub>Δt = Δp. A "quick push" implies a short Δt, meaning the force needs to be relatively large to impart a significant change in momentum.
Momentum: Mass in Motion
Momentum (p) is a measure of an object's mass in motion. It's the product of an object's mass (m) and its velocity (v): p = mv. The quick push changes the block's momentum from zero (initially at rest) to a certain value, determined by the impulse received.
Friction: Opposing the Motion
Once the block is moving, friction plays a significant role in its subsequent motion. Two types of friction are crucial here:
Kinetic Friction: Friction During Motion
Kinetic friction (F<sub>k</sub>) acts to oppose the motion of the block as it slides across the surface. It's directly proportional to the normal force (N) exerted by the surface on the block and depends on the coefficient of kinetic friction (μ<sub>k</sub>), a property of the materials in contact: F<sub>k</sub> = μ<sub>k</sub>N. This frictional force reduces the block's acceleration and eventually brings it to a stop.
Static Friction: Friction at Rest
Before the push, static friction prevents the block from moving. Static friction (F<sub>s</sub>) is the force that must be overcome to initiate motion. It's also proportional to the normal force, but the coefficient of static friction (μ<sub>s</sub>) is generally greater than the coefficient of kinetic friction (μ<sub>s</sub> > μ<sub>k</sub>). The maximum value of static friction (F<sub>s,max</sub> = μ<sub>s</sub>N) must be exceeded for the block to start moving.
Energy Considerations: Work and Energy Transfer
The "quick push" involves the transfer of energy:
Work-Energy Theorem
The work-energy theorem states that the net work done on an object is equal to the change in its kinetic energy (KE): W<sub>net</sub> = ΔKE. The work done by the push increases the block's kinetic energy from zero to a value determined by the force and distance over which the force acts. The work done by friction decreases the block's kinetic energy until it comes to rest.
Kinetic Energy: Energy of Motion
Kinetic energy is the energy an object possesses due to its motion. It's given by: KE = 1/2mv². The greater the velocity, the greater the kinetic energy. The work done by the push converts some of the energy from the pusher into kinetic energy of the block.
Potential Energy and Conservation of Energy
While not directly involved in the initial push, if the block were on an inclined plane, gravitational potential energy would also play a role. The total mechanical energy (sum of kinetic and potential energy) would remain constant in the absence of non-conservative forces like friction. However, friction dissipates energy as heat, rendering the energy conservation principle less straightforward in a real-world scenario with friction.
Factors Influencing the Motion
Several factors affect the block's motion after the push:
- Magnitude of the push: A stronger push imparts a larger impulse and a higher initial velocity.
- Duration of the push: A longer push, even if of the same average force, results in a larger impulse and higher initial velocity.
- Mass of the block: A heavier block requires a greater force or a longer push to achieve the same acceleration.
- Surface roughness: A rougher surface has a higher coefficient of friction, leading to quicker deceleration.
- Shape and orientation of the block: The distribution of mass and the surface area in contact can influence the frictional force.
Analyzing the Motion: A Mathematical Approach
Let's consider a simplified scenario: a block of mass m is pushed horizontally on a flat surface with a constant force F for a time t. Neglecting air resistance, the acceleration a can be calculated using Newton's second law: a = F/m.
The velocity v after the push can be found using the equation: v = at (since it starts from rest). The distance d traveled during the push is: d = 1/2at².
Once the push stops, the only horizontal force acting on the block is kinetic friction. Using Newton's second law again, but this time with the frictional force, we get a<sub>friction</sub> = -μ<sub>k</sub>g, where g is the acceleration due to gravity. The time t<sub>stop</sub> it takes for the block to stop is: t<sub>stop</sub> = v/μ<sub>k</sub>g. The additional distance d<sub>stop</sub> the block travels before stopping is: d<sub>stop</sub> = vt<sub>stop</sub> - 1/2μ<sub>k</sub>gt<sub>stop</sub>².
Beyond the Basics: Advanced Considerations
While the above analysis provides a good foundational understanding, several advanced aspects can be explored:
- Non-constant forces: Real-world pushes are rarely constant; the force might vary during the push, requiring integral calculus for a precise analysis.
- Non-horizontal surfaces: If the surface is inclined, gravitational force components need to be included.
- Rotational motion: If the push is not perfectly aligned with the center of mass, rotational motion will also be involved, adding another layer of complexity.
- Elastic collisions: If the block collides with another object, the principles of elastic or inelastic collisions need to be considered, including conservation of momentum and kinetic energy (in elastic collisions).
Conclusion: A Simple Act, Complex Physics
The seemingly simple act of pushing a block from rest reveals a fascinating interplay of fundamental physics principles. From Newton's laws to impulse, momentum, friction, and energy transfer, this scenario allows for a deep dive into classical mechanics. While a simplified model provides a good starting point, a more realistic analysis involves considering several factors and incorporating more sophisticated mathematical tools. Understanding these principles is crucial in numerous fields, from engineering and robotics to sports science and even everyday life. By analyzing this simple scenario, we gain valuable insights into the fascinating world of physics and its applications in our world.
Latest Posts
Latest Posts
-
Brecht On Theatre The Development Of An Aesthetic
Apr 02, 2025
-
Nova Beyond The Elements Reactions Documentary Questions
Apr 02, 2025
-
Within The Context Of Rcr Social Responsibility Primarily Refers To
Apr 02, 2025
-
General Purpose Applications Include All The Following Except
Apr 02, 2025
-
Dad 220 Module 6 Project One
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about A Block Initially At Rest Is Given A Quick Push . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
