Angle Of Elevation & Depression Trig Worksheet
Onlines
Apr 02, 2025 · 6 min read
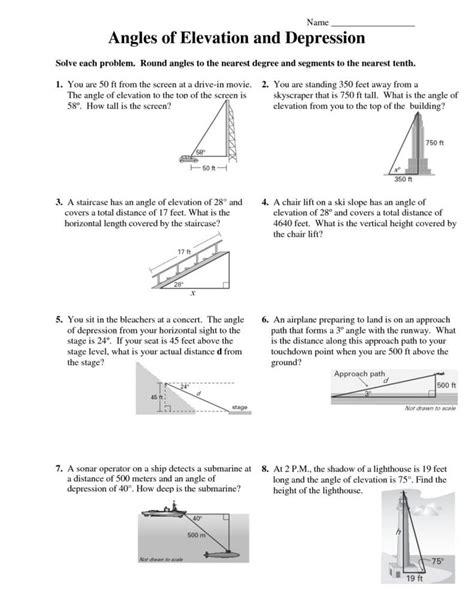
Table of Contents
Angle of Elevation & Depression Trig Worksheet: A Comprehensive Guide
Trigonometry, the study of triangles, offers powerful tools for solving real-world problems involving angles and distances. One particularly useful application is finding unknown distances or heights using angles of elevation and depression. This comprehensive guide will explore the concepts of angles of elevation and depression, provide step-by-step solutions to various problems, and offer tips for mastering trigonometric calculations related to these angles. We’ll be working through a variety of problems similar to those found on a typical trigonometry worksheet.
Understanding Angles of Elevation and Depression
Before diving into problem-solving, let's clarify the terminology:
Angle of Elevation: This is the angle formed between the horizontal line of sight and the line of sight up to an object. Imagine you're looking up at a bird in the sky; the angle between your horizontal gaze and your gaze towards the bird is the angle of elevation.
Angle of Depression: This is the angle formed between the horizontal line of sight and the line of sight down to an object. Think of looking down from a cliff at a boat in the water; the angle between your horizontal gaze and your gaze towards the boat is the angle of depression.
Important Note: Angles of elevation and depression are always measured from the horizontal line of sight. They are not measured from the vertical.
Key Trigonometric Ratios
Solving problems involving angles of elevation and depression requires a solid understanding of the three basic trigonometric ratios:
- Sine (sin): Opposite side / Hypotenuse
- Cosine (cos): Adjacent side / Hypotenuse
- Tangent (tan): Opposite side / Adjacent side
Remember the mnemonic SOH CAH TOA to help you recall these ratios: Sin = Opposite/Hypotenuse, Cos = Adjacent/Hypotenuse, Tan = Opposite/Adjacent.
Sample Problems and Solutions
Let's work through several examples to illustrate the application of angles of elevation and depression in trigonometric problems. These examples cover a range of difficulty levels, mirroring what you might find on a trig worksheet.
Problem 1: The Tall Tree
A surveyor stands 100 feet from the base of a tall tree. The angle of elevation to the top of the tree is 30°. How tall is the tree?
Solution:
-
Draw a diagram: Draw a right-angled triangle with the surveyor's position at one corner, the base of the tree at another corner, and the top of the tree at the third corner. The distance from the surveyor to the tree (100 feet) is the adjacent side, and the height of the tree is the opposite side.
-
Identify the relevant trigonometric ratio: We know the adjacent side and want to find the opposite side. The tangent ratio relates these two: tan(θ) = opposite/adjacent.
-
Substitute the values: tan(30°) = height/100
-
Solve for the height: height = 100 * tan(30°) Using a calculator, tan(30°) ≈ 0.577. Therefore, height ≈ 100 * 0.577 ≈ 57.7 feet.
Therefore, the tree is approximately 57.7 feet tall.
Problem 2: The Airplane's Altitude
An airplane is flying at a constant altitude. A ground observer 1.5 miles from the airplane's takeoff point observes the airplane at an angle of elevation of 25°. What is the airplane's altitude?
Solution:
This problem is similar to the previous one. We use the tangent ratio:
-
Diagram: Draw a right-angled triangle with the observer's position, the takeoff point, and the airplane's current position as the vertices.
-
Ratio: tan(25°) = altitude/1.5 miles
-
Solve: altitude = 1.5 * tan(25°) ≈ 1.5 * 0.466 ≈ 0.7 miles.
Therefore, the airplane's altitude is approximately 0.7 miles.
Problem 3: The Cliffside Observation
A hiker stands on a cliff overlooking the ocean. The angle of depression to a boat is 20°. If the cliff is 150 meters high, how far is the boat from the base of the cliff?
Solution:
Remember that the angle of depression from the hiker to the boat is equal to the angle of elevation from the boat to the hiker (alternate interior angles).
-
Diagram: Draw a right-angled triangle.
-
Ratio: tan(20°) = 150 meters / distance to boat
-
Solve: distance to boat = 150 meters / tan(20°) ≈ 150 / 0.364 ≈ 412 meters.
Therefore, the boat is approximately 412 meters from the base of the cliff.
Problem 4: Two Observers
Two observers are standing 500 meters apart. They both observe a hot air balloon. The angle of elevation from the first observer is 35°, and the angle of elevation from the second observer is 40°. How high is the hot air balloon?
Solution: This problem requires a more advanced approach using two right-angled triangles and some algebraic manipulation.
-
Diagram: Draw two right-angled triangles, one for each observer. Let 'h' be the height of the balloon, 'x' be the distance from the first observer to the point directly below the balloon, and '500-x' be the distance from the second observer to the point directly below the balloon.
-
Equations: We have two equations based on the tangent ratio:
- tan(35°) = h/x
- tan(40°) = h/(500-x)
-
Solve for x: From the first equation, x = h/tan(35°). Substitute this into the second equation: tan(40°) = h/(500 - h/tan(35°)).
-
Solve for h: This equation can be solved algebraically for 'h'. It involves some manipulation, but after solving for h, you get a numerical value for the height of the balloon. This is best solved using a calculator capable of solving algebraic equations or using numerical methods.
Problem 5: The Inclined Plane
A ramp is inclined at an angle of 15° to the horizontal. A box is pushed up the ramp a distance of 10 meters. How high vertically has the box been raised?
Solution:
This problem utilizes the sine function:
-
Diagram: Draw a right-angled triangle representing the ramp.
-
Ratio: sin(15°) = vertical height / 10 meters
-
Solve: vertical height = 10 meters * sin(15°) ≈ 2.59 meters
Therefore, the box has been raised approximately 2.59 meters vertically.
Tips for Mastering Angle of Elevation and Depression Problems
-
Always draw a diagram: A well-labeled diagram is crucial for visualizing the problem and identifying the relevant sides and angles.
-
Identify the known and unknown quantities: Clearly state what you know (angles, distances) and what you need to find.
-
Choose the correct trigonometric ratio: Carefully select the ratio (sin, cos, tan) that relates the known and unknown quantities.
-
Use a calculator: Be sure to use a calculator that is capable of handling trigonometric functions. Ensure your calculator is set to the correct angle mode (degrees or radians).
-
Check your answer: Does your answer make sense in the context of the problem? Is the height or distance reasonable?
Advanced Applications and Further Exploration
The concepts of angles of elevation and depression extend far beyond simple textbook problems. They have numerous applications in fields such as:
- Surveying: Determining heights of buildings, mountains, and other structures.
- Navigation: Calculating distances and bearings at sea or in the air.
- Engineering: Designing structures and ensuring stability.
- Astronomy: Measuring distances to celestial objects.
- Ballistics: Calculating the trajectory of projectiles.
By mastering the fundamental principles outlined in this guide, you’ll be well-equipped to tackle more complex problems involving angles of elevation and depression. Remember consistent practice is key to building proficiency in trigonometry and developing a deeper understanding of its applications in the real world. Continue practicing with different types of problems and scenarios to enhance your problem-solving skills and confidence in tackling trigonometry worksheets effectively.
Latest Posts
Latest Posts
-
A Recommended Procedure Regarding Decontamination Is To
Apr 03, 2025
-
Medical Terminology Crossword Puzzle Answer Key
Apr 03, 2025
-
The Paper Is Stating The Poems Summaries Themes Topics Transitions
Apr 03, 2025
-
Hw 7 1 1 3 Arithmetic And Geometric Sequences
Apr 03, 2025
-
A Client With Copd Has A Blood Ph Of 7 25
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Angle Of Elevation & Depression Trig Worksheet . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
