Chapter 21 Temperature Heat And Expansion Answer Key
Onlines
Mar 30, 2025 · 6 min read
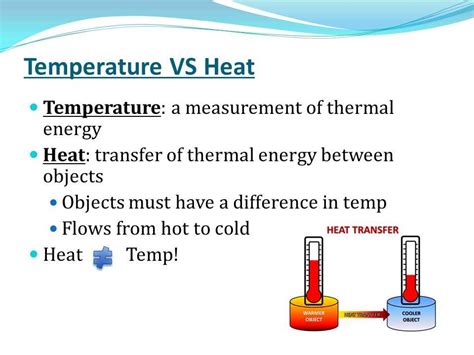
Table of Contents
Chapter 21: Temperature, Heat, and Expansion – A Comprehensive Guide
This comprehensive guide delves into the intricacies of Chapter 21, focusing on temperature, heat, and thermal expansion. We'll explore key concepts, provide detailed explanations, and offer practical examples to solidify your understanding. This isn't just an answer key; it's a journey through the fascinating world of thermodynamics.
Understanding Temperature and Heat
Before we dive into specific problems and solutions, let's establish a firm foundation in the fundamental concepts.
What is Temperature?
Temperature is a measure of the average kinetic energy of the particles within a substance. Higher temperature indicates particles are moving faster and possess more kinetic energy. It's crucial to distinguish between temperature and heat. They are related but distinct concepts.
The Difference Between Heat and Temperature
Heat, on the other hand, is the transfer of thermal energy between objects at different temperatures. Heat flows spontaneously from a hotter object to a colder one until thermal equilibrium is reached – meaning both objects reach the same temperature. Think of it like this: temperature is a measure of how hot something is, while heat is the energy transferred due to a temperature difference.
Measuring Temperature
Various scales are used to measure temperature, most notably:
- Celsius (°C): Based on the freezing (0°C) and boiling (100°C) points of water at standard atmospheric pressure.
- Fahrenheit (°F): Another common scale, with water freezing at 32°F and boiling at 212°F.
- Kelvin (K): The absolute temperature scale, where 0 K represents absolute zero – the theoretical point where all molecular motion ceases. Kelvin is used extensively in scientific calculations.
Conversion Between Temperature Scales
You'll often need to convert between these scales. Here are the essential formulas:
- Celsius to Fahrenheit: °F = (9/5)°C + 32
- Fahrenheit to Celsius: °C = (5/9)(°F - 32)
- Celsius to Kelvin: K = °C + 273.15
- Kelvin to Celsius: °C = K - 273.15
Thermal Expansion
When a substance is heated, its particles gain kinetic energy and move further apart, resulting in an increase in volume. This phenomenon is known as thermal expansion. The degree of expansion varies depending on the material and the temperature change.
Types of Thermal Expansion
-
Linear Expansion: Describes the change in length of a solid when heated or cooled. The formula is: ΔL = αL₀ΔT, where ΔL is the change in length, α is the coefficient of linear expansion (material-specific constant), L₀ is the original length, and ΔT is the change in temperature.
-
Area Expansion: Accounts for the change in area of a material due to temperature changes. The formula is more complex but generally involves a coefficient of area expansion, which is approximately twice the coefficient of linear expansion.
-
Volume Expansion: Deals with the change in volume of a substance, particularly liquids and gases. The formula is: ΔV = βV₀ΔT, where ΔV is the change in volume, β is the coefficient of volume expansion, V₀ is the initial volume, and ΔT is the change in temperature. For gases, under constant pressure, the coefficient of volume expansion is approximately 1/273.15 per degree Celsius.
Specific Heat Capacity
Specific heat capacity (c) is a measure of the amount of heat required to raise the temperature of 1 kilogram of a substance by 1 degree Celsius (or 1 Kelvin). Different materials have different specific heat capacities. Water, for instance, has a relatively high specific heat capacity, meaning it can absorb a significant amount of heat with a relatively small temperature increase.
The Specific Heat Equation
The relationship between heat (Q), mass (m), specific heat capacity (c), and temperature change (ΔT) is given by:
Q = mcΔT
This equation is fundamental to solving many problems related to heat transfer.
Heat Transfer Mechanisms
Heat can be transferred through three primary mechanisms:
-
Conduction: Heat transfer through direct contact within a material. Metals are good conductors, while insulators like wood are poor conductors.
-
Convection: Heat transfer through the movement of fluids (liquids or gases). Convection currents are responsible for distributing heat in many natural phenomena.
-
Radiation: Heat transfer through electromagnetic waves. The sun's warmth reaching Earth is an example of radiation.
Solving Problems Related to Temperature, Heat, and Expansion
Now, let's tackle some example problems to illustrate the application of these principles. Remember to always identify the known variables and the unknown variable you need to solve for. Properly utilizing the formulas discussed above is key.
Example Problem 1: Linear Expansion
A steel bridge is 1000 meters long at 20°C. What will its length be at 40°C if the coefficient of linear expansion for steel is 12 x 10⁻⁶ /°C?
Solution:
We use the linear expansion formula: ΔL = αL₀ΔT
- α = 12 x 10⁻⁶ /°C
- L₀ = 1000 m
- ΔT = 40°C - 20°C = 20°C
ΔL = (12 x 10⁻⁶ /°C)(1000 m)(20°C) = 0.24 m
The new length of the bridge will be 1000 m + 0.24 m = 1000.24 m
Example Problem 2: Specific Heat
How much heat is required to raise the temperature of 2 kg of water from 20°C to 80°C? The specific heat capacity of water is approximately 4186 J/kg°C.
Solution:
We use the specific heat equation: Q = mcΔT
- m = 2 kg
- c = 4186 J/kg°C
- ΔT = 80°C - 20°C = 60°C
Q = (2 kg)(4186 J/kg°C)(60°C) = 502,320 J
Example Problem 3: Volume Expansion
A glass bottle has a volume of 1 liter at 25°C. If the coefficient of volume expansion for glass is 27 x 10⁻⁶ /°C, what will its volume be at 50°C?
Solution:
We use the volume expansion formula: ΔV = βV₀ΔT
- β = 27 x 10⁻⁶ /°C
- V₀ = 1 L = 1000 cm³
- ΔT = 50°C - 25°C = 25°C
ΔV = (27 x 10⁻⁶ /°C)(1000 cm³)(25°C) = 0.675 cm³
The new volume will be 1000 cm³ + 0.675 cm³ = 1000.675 cm³
Further Exploration
This guide provides a solid foundation in the concepts of temperature, heat, and expansion. To further enhance your understanding, explore more complex topics such as:
- Thermodynamics: The study of heat and its relationship to other forms of energy.
- Heat Engines: Devices that convert heat energy into mechanical work.
- Refrigeration and Air Conditioning: Applications of thermodynamics for controlling temperature.
- Phase Transitions: Changes in the state of matter (solid, liquid, gas).
By mastering these fundamental concepts and practicing problem-solving, you'll develop a strong understanding of Chapter 21 and its applications in various fields of science and engineering. Remember to consult your textbook and other resources for additional practice problems and in-depth explanations. This guide is a stepping stone to a deeper comprehension of this fascinating subject. Keep exploring, keep learning!
Latest Posts
Latest Posts
-
Surface Area Of Solids Using Nets Worksheet Answer Key
Apr 01, 2025
-
Investigation Dna Proteins And Mutations Worksheet
Apr 01, 2025
-
Quotes From Stranger In A Strange Land
Apr 01, 2025
-
This Is A 2 Page Document Answer Key
Apr 01, 2025
-
Give Me Liberty Chapter 16 Notes
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Chapter 21 Temperature Heat And Expansion Answer Key . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
