Circuit Training Calculus First Half Review
Onlines
Apr 07, 2025 · 6 min read
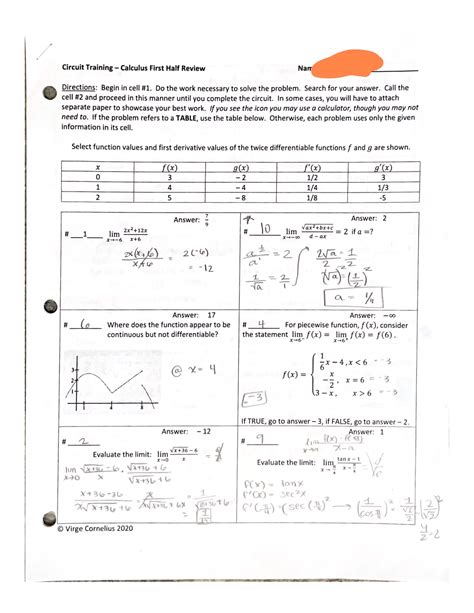
Table of Contents
Circuit Training Calculus: First Half Review – Conquer Your Calculus Fears!
Are you feeling overwhelmed by the sheer volume of information covered in the first half of your calculus course? Do you find yourself struggling to connect concepts and apply them effectively to problem-solving? Don't worry, you're not alone! Many students find the initial stages of calculus challenging. This comprehensive review will use a circuit training approach to help you solidify your understanding and build a strong foundation for the rest of the course. We'll cover key topics, provide practice problems, and offer strategies for mastering calculus.
Section 1: Functions and Their Properties
This section revisits the fundamental building blocks of calculus: functions. A solid grasp of functions is crucial for understanding derivatives and integrals.
1.1 Function Notation and Domains
Remember those pesky domain restrictions? Let's refresh our understanding of function notation (f(x), g(x), etc.) and how to determine the domain of a function, considering restrictions like square roots and denominators.
Example: Find the domain of f(x) = √(x - 4) / (x² - 9).
- The expression under the square root must be non-negative: x - 4 ≥ 0 => x ≥ 4
- The denominator cannot be zero: x² - 9 ≠ 0 => x ≠ ±3
Therefore, the domain of f(x) is [4, ∞) excluding x = 3.
1.2 Function Transformations
Understanding how transformations affect a graph is vital for visualizing functions and their derivatives. Let's review horizontal and vertical shifts, stretches, and reflections.
Example: Describe the transformation of g(x) = 2f(x - 3) + 1, given the graph of f(x).
g(x) represents a vertical stretch by a factor of 2, a horizontal shift to the right by 3 units, and a vertical shift upward by 1 unit.
1.3 Function Composition and Inverses
Composing functions (f(g(x))) and finding inverse functions (f⁻¹(x)) are essential skills needed for later calculus concepts.
Example: Find the composition f(g(x)) and the inverse f⁻¹(x) if f(x) = x² + 1 and g(x) = x - 2.
Section 2: Limits and Continuity
Limits and continuity form the theoretical bedrock of calculus. Understanding limits is essential for defining derivatives.
2.1 Evaluating Limits
Review the techniques for evaluating limits, including direct substitution, factoring, rationalizing, and L'Hôpital's Rule (if you've covered it). Remember to always check for indeterminate forms (0/0, ∞/∞).
Example: Evaluate lim (x→2) (x² - 4) / (x - 2).
This limit can be evaluated by factoring the numerator: lim (x→2) (x - 2)(x + 2) / (x - 2) = lim (x→2) (x + 2) = 4
2.2 Continuity
Recall the definition of continuity: a function is continuous at a point if the limit exists, the function value exists, and they are equal. Identify types of discontinuities (removable, jump, infinite).
Example: Determine if f(x) = |x| is continuous at x = 0.
2.3 Limits at Infinity
Understanding the behavior of functions as x approaches infinity or negative infinity is crucial for analyzing asymptotic behavior.
Example: Find lim (x→∞) (3x² - 2x + 1) / (x² + 5).
Section 3: Derivatives
Derivatives measure the instantaneous rate of change of a function. This section will review various differentiation techniques.
3.1 Definition of the Derivative
Remember the formal definition of the derivative using limits: f'(x) = lim (h→0) [f(x + h) - f(x)] / h. Understanding this definition is fundamental.
Example: Use the definition of the derivative to find the derivative of f(x) = x².
3.2 Basic Differentiation Rules
Master the power rule, constant multiple rule, sum/difference rule, and product/quotient rules. These are the bread and butter of differentiation.
Example: Differentiate f(x) = 3x⁴ - 2x² + 5x - 7.
3.3 Chain Rule
The chain rule is essential for differentiating composite functions. Practice applying the chain rule to various functions.
Example: Differentiate f(x) = (x² + 1)⁵.
3.4 Implicit Differentiation
Learn how to differentiate implicitly defined functions. This is particularly useful when it's difficult or impossible to solve for y explicitly.
Example: Find dy/dx if x² + y² = 25.
3.5 Derivatives of Trigonometric, Exponential, and Logarithmic Functions
Review the derivatives of trigonometric functions (sin x, cos x, tan x, etc.), exponential functions (eˣ), and logarithmic functions (ln x).
Example: Differentiate f(x) = eˣ sin x.
3.6 Higher-Order Derivatives
Understand the concept of higher-order derivatives (second derivative, third derivative, etc.) and their interpretations.
Example: Find the second derivative of f(x) = x³ - 4x² + 7x - 2.
Section 4: Applications of Derivatives
This section applies the derivative concepts to various real-world problems.
4.1 Related Rates
Related rates problems involve finding the rate of change of one quantity in terms of the rate of change of another quantity.
Example: A ladder is leaning against a wall. If the bottom of the ladder is sliding away from the wall at a certain rate, find the rate at which the top of the ladder is sliding down.
4.2 Optimization Problems
Optimization problems involve finding the maximum or minimum value of a function within a given interval.
Example: Find the dimensions of a rectangle with a fixed perimeter that maximizes its area.
4.3 Curve Sketching
Use derivatives to analyze the behavior of a function, including increasing/decreasing intervals, concavity, inflection points, and asymptotes. This helps in accurately sketching the graph.
Example: Sketch the graph of f(x) = x³ - 3x² + 2.
4.4 Mean Value Theorem
The Mean Value Theorem states that there exists a point within an interval where the instantaneous rate of change equals the average rate of change.
Example: Apply the Mean Value Theorem to f(x) = x² on the interval [1, 3].
Section 5: Circuit Training Exercises
Now, let's put your knowledge to the test with a series of exercises, structured as a circuit training session:
Round 1: Quick Fire Differentiation
- Differentiate f(x) = 5x³ - 2x + 7
- Differentiate g(x) = sin(2x)
- Differentiate h(x) = e^(x²)
- Differentiate k(x) = ln(x + 1)
- Differentiate m(x) = (x² + 3x) / (x - 1)
Round 2: Limit Challenges
- Evaluate lim (x→0) (sin x) / x
- Evaluate lim (x→∞) (2x² - 3x + 1) / (x² + 2)
- Evaluate lim (x→1) (x² - 1) / (x - 1)
- Evaluate lim (x→0) (e^x - 1) / x
- Determine if f(x) = 1/x is continuous at x = 0.
Round 3: Application Problems
- A balloon is rising vertically at a constant rate. Find the rate at which the distance between the balloon and an observer on the ground is changing at a specific moment.
- Find the maximum area of a rectangle that can be inscribed in a circle with radius r.
- Sketch the graph of f(x) = x⁴ - 4x³.
Round 4: Mixed Bag
- Find the domain of f(x) = √(x² - 4)
- Find the inverse of f(x) = 2x + 1
- Use implicit differentiation to find dy/dx if x³ + y³ = 3xy.
- Apply the Mean Value Theorem to f(x) = x³ on the interval [0, 2].
- Find the second derivative of f(x) = ln(x).
This circuit training approach allows you to practice a variety of problems across different topics, solidifying your understanding and building confidence. Remember to check your solutions and review the concepts you found challenging.
Conclusion:
This comprehensive review utilizes a circuit training methodology to reinforce your grasp of fundamental calculus concepts. By consistently practicing a range of problem types, you'll effectively hone your skills and develop a deeper understanding. This structured approach will empower you to tackle the second half of your calculus course with confidence and achieve academic success! Remember to consult your textbook, lecture notes, and seek help from your instructor or peers when needed. Good luck conquering calculus!
Latest Posts
Latest Posts
-
The Accompanying Graph Represents Haydens Fro Yo
Apr 09, 2025
-
With A Few Exceptions When Is Lockout Tagout Required
Apr 09, 2025
-
Label The Structures Within One Lobe Of The Kidney
Apr 09, 2025
-
2020 Practice Exam 3 Mcq Ap Bio
Apr 09, 2025
-
6 3 Skills Practice Tests For Parallelograms
Apr 09, 2025
Related Post
Thank you for visiting our website which covers about Circuit Training Calculus First Half Review . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.