Constant Velocity Particle Model Worksheet 4
Onlines
Apr 05, 2025 · 6 min read
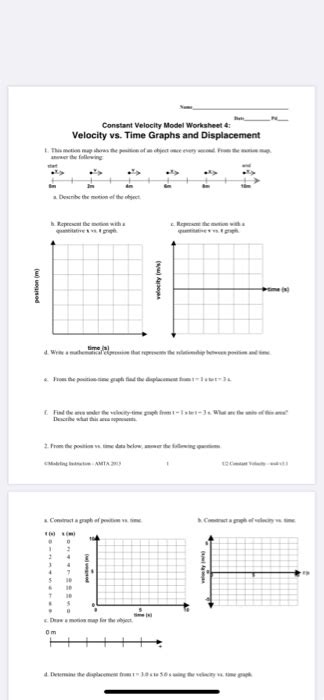
Table of Contents
Constant Velocity Particle Model: Worksheet 4 Deep Dive
This article serves as a comprehensive guide to understanding and mastering the concepts within a hypothetical "Worksheet 4" focused on the constant velocity particle model. While a specific worksheet doesn't exist publicly, we'll cover all the key principles, problem-solving techniques, and advanced applications related to this physics topic. We'll delve deep into the core concepts, offering numerous examples and practice problems to solidify your understanding.
What is the Constant Velocity Particle Model?
The constant velocity particle model is a simplification used in physics to analyze the motion of objects. It assumes that:
- The object is a particle: This means we ignore the object's size and shape, treating it as a single point in space.
- The velocity is constant: The object's speed and direction remain unchanged throughout the motion. This implies zero acceleration.
This model is incredibly useful for introductory physics problems because it reduces the complexity of real-world scenarios. While it's an idealization (most real-world objects experience some acceleration), it provides a solid foundation for understanding more complex motion later on.
Key Concepts and Formulas:
Several core concepts underpin the constant velocity particle model:
-
Displacement (Δx): The change in position of the particle. It's a vector quantity, meaning it has both magnitude (distance) and direction. Δx = x<sub>f</sub> - x<sub>i</sub>, where x<sub>f</sub> is the final position and x<sub>i</sub> is the initial position.
-
Velocity (v): The rate of change of displacement. For constant velocity, it's simply the displacement divided by the time interval. It's also a vector quantity. v = Δx / Δt, where Δt is the change in time.
-
Time (Δt): The duration of the motion.
-
Distance: The total length of the path traveled. In the case of constant velocity, distance is equal to the magnitude of the displacement.
Common Problem Types and Solution Strategies:
Worksheet 4, in a hypothetical context, would likely feature a variety of problem types. Let's explore some common scenarios and their solutions:
1. Calculating Displacement:
-
Problem: A car travels at a constant velocity of 20 m/s east for 5 seconds. What is its displacement?
-
Solution: We use the formula v = Δx / Δt. Rearranging, we get Δx = v * Δt = (20 m/s) * (5 s) = 100 m east.
2. Calculating Velocity:
-
Problem: A runner covers a distance of 100 meters in 10 seconds. Assuming constant velocity, what is the runner's velocity?
-
Solution: Again, we use v = Δx / Δt. In this case, Δx = 100 m and Δt = 10 s. Therefore, v = 100 m / 10 s = 10 m/s. The direction isn't explicitly stated, so we can only give the magnitude of the velocity.
3. Calculating Time:
-
Problem: A plane flies at a constant velocity of 500 km/h north for a displacement of 1500 km. How long does the flight take?
-
Solution: We rearrange the velocity formula to solve for time: Δt = Δx / v = 1500 km / 500 km/h = 3 hours.
4. Problems Involving Multiple Segments:
These problems involve an object moving with constant velocity in different directions or at different speeds.
-
Problem: A cyclist travels 5 km east at 15 km/h, then turns and travels 10 km north at 20 km/h. Calculate the total time of the journey and the final displacement from the starting point.
-
Solution: This problem requires breaking it down into segments:
- Segment 1 (East): Time = Distance / Velocity = 5 km / 15 km/h = 1/3 hour.
- Segment 2 (North): Time = Distance / Velocity = 10 km / 20 km/h = 1/2 hour.
- Total Time: 1/3 hour + 1/2 hour = 5/6 hour.
To find the final displacement, we use the Pythagorean theorem since the segments are at right angles:
- Displacement = √((5 km)² + (10 km)²) ≈ 11.2 km. The direction can be found using trigonometry (arctan(10/5) ≈ 63.4° north of east).
5. Problems with Relative Velocity:
Relative velocity considers the velocity of an object relative to another object or frame of reference.
-
Problem: A boat travels at 10 m/s relative to the water, and the river flows at 5 m/s. What is the boat's velocity relative to the riverbank if it's traveling downstream? Upstream?
-
Solution:
- Downstream: The velocities add up: 10 m/s + 5 m/s = 15 m/s.
- Upstream: The velocities subtract: 10 m/s - 5 m/s = 5 m/s.
Advanced Applications and Considerations:
While simple, the constant velocity model can be used to introduce more advanced concepts:
-
Vectors: Displacement and velocity are vector quantities, requiring consideration of both magnitude and direction. This leads into the use of vector addition and subtraction.
-
Graphs: Position-time graphs for constant velocity motion are straight lines, with the slope representing the velocity. Understanding these graphs is crucial.
-
Limitations: It's vital to understand that this model is a simplification. Real-world objects rarely maintain perfectly constant velocity. Factors like friction, air resistance, and changing forces will cause accelerations. This model is a starting point for understanding more complex models.
-
Coordinate Systems: Problems often require setting up a coordinate system to define positions and directions.
Practice Problems (Hypothetical Worksheet 4):
Here are some sample problems that might appear on a hypothetical "Worksheet 4":
-
A train travels at a constant speed of 60 km/h for 2 hours. What is its displacement?
-
A ball rolls across a flat surface at 2 m/s for 5 seconds before stopping. What was its average velocity? (Note: This introduces the concept of average velocity even within the constant velocity context – the instantaneous velocity is constant, but when it stops, the average velocity decreases.)
-
A bird flies 100 meters north, then 50 meters east. If the total flight took 20 seconds, what was the bird's average velocity (magnitude and direction)?
-
Two cars are traveling in opposite directions on a highway. Car A is moving at 70 km/h and Car B is moving at 80 km/h. What is the relative velocity of Car A with respect to Car B?
-
A rocket launches vertically at a constant velocity of 1000 m/s. After 10 seconds, what is its altitude? (Introduce the concept of simple vertical motion).
Conclusion:
Mastering the constant velocity particle model is a fundamental step in understanding mechanics. By thoroughly grasping the key concepts, formulas, and problem-solving strategies outlined above, you'll build a robust foundation for tackling more complex physics problems involving acceleration and other forces. Remember to always consider the limitations of the model and its applications in various real-world scenarios. This deep dive should equip you to tackle any challenge presented in a "Worksheet 4" – or any similar assessment – on this important physics topic. Practice consistently, and you'll see significant improvement in your understanding and problem-solving abilities.
Latest Posts
Latest Posts
-
9 2 5 Detect Open Ports With Nmap
Apr 06, 2025
-
Which Statement Is True About Cigna Dental Benefits
Apr 06, 2025
-
A Woodworking Artist Makes Two Types
Apr 06, 2025
-
A Hammer Handle Can Be Damaged When Nailing Due To
Apr 06, 2025
-
Cell Transport Amoeba Sisters Answer Key
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about Constant Velocity Particle Model Worksheet 4 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
