Superposition And Reflection Of Pulses Homework Answers
Onlines
Apr 03, 2025 · 7 min read
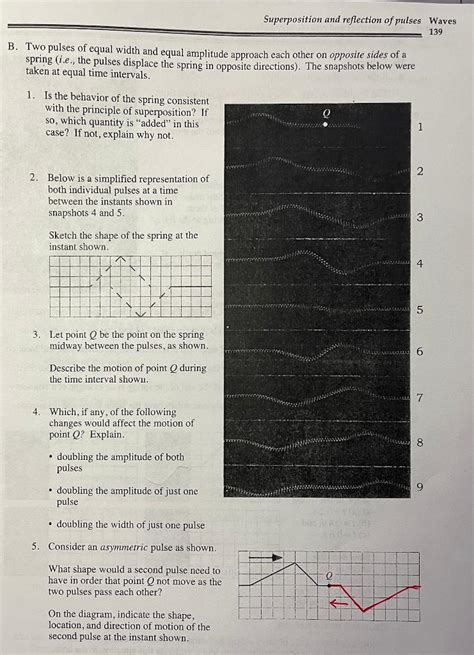
Table of Contents
Superposition and Reflection of Pulses: A Comprehensive Guide
Understanding superposition and reflection of pulses is crucial in mastering wave phenomena in physics. This comprehensive guide delves into these concepts, providing detailed explanations, worked examples, and addressing common homework problems. We'll explore both theoretical underpinnings and practical applications, ensuring a thorough grasp of these essential principles.
What is Superposition?
The principle of superposition states that when two or more waves overlap in the same medium, the resultant displacement at any point is the algebraic sum of the individual displacements caused by each wave. This principle applies to both transverse waves (like waves on a string) and longitudinal waves (like sound waves). Crucially, it assumes that the waves don't significantly affect each other's propagation; this is a valid assumption for many wave systems, particularly those with small amplitudes.
Key Implications of Superposition:
- Constructive Interference: When waves overlap in phase (crests aligning with crests, troughs with troughs), their amplitudes add together, resulting in a larger amplitude wave. This is known as constructive interference.
- Destructive Interference: When waves overlap out of phase (crests aligning with troughs), their amplitudes subtract, resulting in a smaller amplitude wave, or even cancellation if the amplitudes are equal. This is destructive interference.
- Linearity: Superposition is a direct consequence of the linearity of the wave equation. This means that if you have two solutions to the wave equation, their sum is also a solution.
Examples of Superposition:
- Ripples in a pond: When two stones are dropped into a pond, the overlapping ripples demonstrate superposition. In areas where crests overlap, larger ripples form (constructive interference), while in areas where crests and troughs overlap, the ripples may cancel out (destructive interference).
- Sound waves: When two musical instruments play simultaneously, the sound waves superpose. The resulting sound is a combination of the individual sounds, with constructive and destructive interference affecting the overall loudness and timbre.
Reflection of Pulses
When a wave encounters a boundary, it undergoes reflection. The nature of the reflection depends on the boundary conditions. We'll focus on two primary types:
-
Fixed End Reflection: When a wave pulse traveling on a string encounters a fixed end (e.g., the string is tied to a wall), it reflects inverted. This means that a crest reflects as a trough, and vice-versa. The amplitude of the reflected pulse is typically the same as the incident pulse (assuming no energy loss).
-
Free End Reflection: When a wave pulse encounters a free end (e.g., the string is free to move up and down), it reflects upright. A crest reflects as a crest, and a trough reflects as a trough. Again, the amplitude typically remains the same.
Factors Affecting Reflection:
- Impedance Mismatch: The reflection coefficient, which determines the amplitude of the reflected wave, is influenced by the impedance mismatch at the boundary. A large impedance mismatch leads to a larger reflected wave. Impedance is a measure of how much the medium resists the propagation of waves.
- Energy Conservation: The total energy of the system is conserved during reflection. Some energy might be lost due to friction or absorption, but ideally, the sum of the energies of the incident and reflected waves equals the energy of the incident wave.
Superposition and Reflection Combined:
Many real-world scenarios involve both superposition and reflection simultaneously. For example, consider a wave pulse traveling on a string that's fixed at one end. When the pulse reaches the fixed end, it reflects inverted. The incident and reflected pulses then superpose, creating complex interference patterns.
Let's analyze a few scenarios step-by-step:
Scenario 1: Two identical pulses approaching each other on a string, fixed at both ends.
- Initial State: Two identical positive pulses are traveling towards each other.
- Collision: The pulses overlap, undergoing superposition. At the point of maximum overlap, the resultant displacement is twice the amplitude of each individual pulse (constructive interference).
- Post-Collision: After overlapping, the pulses continue to travel in their original directions, maintaining their original shapes and amplitudes. This is because the pulses don't affect each other's propagation permanently.
Scenario 2: A single pulse traveling on a string fixed at one end.
- Incident Pulse: A positive pulse travels towards the fixed end.
- Reflection: Upon reaching the fixed end, the pulse reflects, inverting to become a negative pulse.
- Superposition: The incident and reflected pulses now superpose. Initially, there will be destructive interference near the fixed end, potentially leading to a moment of zero displacement. The further away from the fixed end, the less the interference will affect the overall shape of the pulse.
- Continued Propagation: Once the reflected pulse has completely separated from the original pulse, they continue their individual journeys.
Scenario 3: A pulse on a string with a change in density (impedance mismatch).
In this scenario, the string's density changes abruptly at a point. This creates an impedance mismatch, leading to both reflection and transmission of the pulse. Part of the pulse will be reflected (with a possible inversion, depending on the change in density), and part will be transmitted (potentially with a change in amplitude). The superposition of the incident, reflected, and transmitted waves determines the overall wave pattern.
Solving Homework Problems: A Step-by-Step Approach
Let's tackle a few typical homework problems that combine superposition and reflection:
Problem 1: A positive pulse of amplitude A is traveling on a string fixed at both ends. Sketch the shape of the string at various times as the pulse travels and reflects.
Solution:
- Initial State: Sketch the pulse traveling towards one end.
- First Reflection: At the fixed end, the pulse reflects, inverting to become a negative pulse of amplitude -A. Sketch this reflected pulse.
- Superposition (during reflection): Sketch the superposition of the incident and reflected pulses. There will be a region of destructive interference near the fixed end.
- After Reflection: After the pulses completely separate, sketch both pulses continuing in their respective directions.
- Second Reflection: The reflected negative pulse will travel towards the other fixed end, again reflecting and inverting, thus becoming a positive pulse. Repeat steps 2-4 for this second reflection.
- Continued Reflections: Continue this process, showing the multiple reflections and superpositions.
Problem 2: Two pulses, one positive (amplitude A) and one negative (amplitude -A/2), are traveling towards each other on a string. Sketch the resultant waveform at various times before, during, and after the pulses overlap.
Solution:
- Before Overlap: Sketch the two pulses moving towards each other.
- Partial Overlap: As the pulses start to overlap, sketch the superposition at several time steps. The amplitude of the superposition will be A - A/2 = A/2 in the region of overlap.
- Maximum Overlap: At maximum overlap, the resultant amplitude will be A – A/2 = A/2 throughout the region of the smaller pulse.
- After Overlap: As the pulses separate, sketch the resultant waveforms. The pulses will retain their original shapes and amplitudes.
Problem 3: A pulse travels from a lighter string to a heavier string. Describe the reflection and transmission.
Solution: Since the pulse goes from a lighter (lower impedance) to a heavier (higher impedance) string, a portion of the pulse will be reflected back into the lighter string inverted, and a portion will be transmitted into the heavier string, with reduced amplitude and speed, but without inversion. The amplitudes of the reflected and transmitted pulses will depend on the specific impedance mismatch.
Advanced Concepts and Applications
The principles of superposition and reflection extend far beyond simple string pulses. They are fundamental to understanding:
- Interference patterns in optics: Young's double-slit experiment beautifully demonstrates superposition and interference of light waves.
- Standing waves: These are formed by the superposition of two waves traveling in opposite directions with the same frequency and amplitude.
- Acoustic resonance: The superposition of sound waves can create resonant frequencies in musical instruments and other systems.
- Seismic wave propagation: Superposition and reflection play critical roles in understanding how seismic waves propagate through the Earth's layers.
- Quantum mechanics: Superposition is a cornerstone of quantum mechanics, where particles can exist in multiple states simultaneously.
By mastering the fundamental concepts of superposition and reflection, you gain a powerful tool for understanding a wide range of wave phenomena. Thorough practice with various problems, like those outlined above, is key to building intuition and solving more complex scenarios. Remember to always consider the boundary conditions and the principle of energy conservation when analyzing wave behavior.
Latest Posts
Latest Posts
-
What Type Of Current Is Illustrated In The Diagram Below
Apr 04, 2025
-
The Land Ethic Aldo Leopold Summary
Apr 04, 2025
-
A Farewell To Arms Quotes With Page Numbers
Apr 04, 2025
-
Which Disorder Would The Nurse Classify As Neurodevelopmental
Apr 04, 2025
-
Loss Grief Death Hesi Case Study
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Superposition And Reflection Of Pulses Homework Answers . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
