Unit 1 Kinematics 1.m Projectile Motion Answer Key
Onlines
Mar 29, 2025 · 5 min read
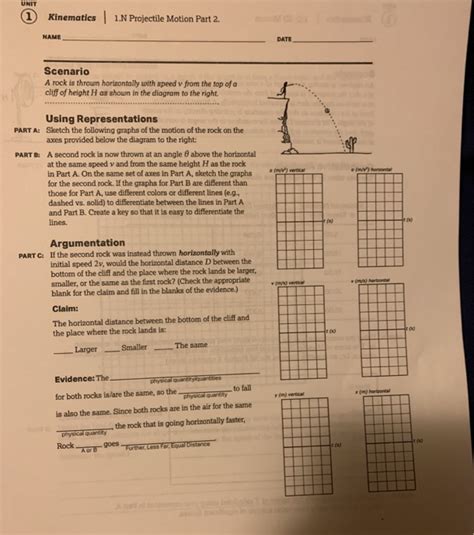
Table of Contents
Unit 1 Kinematics 1.M Projectile Motion: A Comprehensive Guide
This comprehensive guide delves into the intricacies of projectile motion, a fundamental concept within the realm of kinematics. We'll explore the key principles, equations, and problem-solving techniques necessary for a thorough understanding of this topic. This guide serves as an extensive resource, aiming to be more than just an "answer key," providing a deep dive into the underlying physics and offering practical applications.
What is Projectile Motion?
Projectile motion describes the motion of an object launched into the air, subject only to the force of gravity (neglecting air resistance). Understanding projectile motion is crucial in various fields, including sports science, engineering, and military applications. Key characteristics include:
- Parabolic Trajectory: The path followed by a projectile is a parabola. This is due to the constant downward acceleration caused by gravity.
- Independent Horizontal and Vertical Motion: The horizontal and vertical components of motion are independent of each other. This means the horizontal velocity remains constant (assuming no air resistance), while the vertical velocity changes due to gravity.
- Constant Horizontal Velocity: In the absence of air resistance, the horizontal velocity of a projectile remains constant throughout its flight.
- Constant Vertical Acceleration: The vertical acceleration of a projectile is always equal to the acceleration due to gravity (approximately 9.8 m/s² downwards).
Key Equations for Projectile Motion:
Several key equations govern projectile motion. These equations allow us to calculate various parameters, including time of flight, range, maximum height, and velocity at any point in the trajectory. Let's break down the most important ones:
Horizontal Motion:
- x = v₀ₓt where:
- x = horizontal displacement
- v₀ₓ = initial horizontal velocity
- t = time
Vertical Motion:
-
vᵧ = v₀ᵧ - gt where:
- vᵧ = final vertical velocity
- v₀ᵧ = initial vertical velocity
- g = acceleration due to gravity (approximately 9.8 m/s²)
- t = time
-
y = v₀ᵧt - (1/2)gt² where:
- y = vertical displacement
- v₀ᵧ = initial vertical velocity
- g = acceleration due to gravity (approximately 9.8 m/s²)
- t = time
-
vᵧ² = v₀ᵧ² - 2gy where:
- vᵧ = final vertical velocity
- v₀ᵧ = initial vertical velocity
- g = acceleration due to gravity (approximately 9.8 m/s²)
- y = vertical displacement
Understanding the Components of Initial Velocity:
The initial velocity (v₀) is often given as a magnitude and an angle (θ) above the horizontal. We can resolve this into its horizontal (v₀ₓ) and vertical (v₀ᵧ) components using trigonometry:
- v₀ₓ = v₀cosθ
- v₀ᵧ = v₀sinθ
Solving Projectile Motion Problems: A Step-by-Step Approach
Solving projectile motion problems often involves a systematic approach. Here's a breakdown of the steps involved:
-
Draw a Diagram: Always start by drawing a clear diagram illustrating the problem. Include the initial velocity, angle of projection, and any other relevant information.
-
Resolve the Initial Velocity: Break down the initial velocity into its horizontal and vertical components using trigonometry.
-
Identify Knowns and Unknowns: List all the known quantities (initial velocity, angle, etc.) and the unknowns you need to find (time of flight, range, maximum height, etc.).
-
Choose the Appropriate Equations: Select the relevant equations based on the knowns and unknowns. You'll likely use a combination of the equations listed above.
-
Solve for the Unknowns: Use algebraic manipulation to solve for the unknown quantities. Remember to use consistent units throughout your calculations.
-
Check Your Answer: Always review your answer to ensure it's reasonable and consistent with the problem's context.
Common Projectile Motion Scenarios and Their Solutions:
Let's explore some common scenarios and demonstrate the application of the equations and problem-solving steps:
Scenario 1: Finding Time of Flight
A projectile is launched with an initial velocity of 20 m/s at an angle of 30° above the horizontal. Find the time of flight.
- Solution:
- v₀ = 20 m/s, θ = 30°
- v₀ₓ = v₀cosθ = 20cos30° ≈ 17.32 m/s
- v₀ᵧ = v₀sinθ = 20sin30° = 10 m/s
- At the highest point, vᵧ = 0. Using vᵧ = v₀ᵧ - gt, we can find the time to reach the highest point:
- 0 = 10 - 9.8t => t ≈ 1.02 s
- The total time of flight is twice this time (since the projectile takes the same time to go up and come down):
- Total time of flight ≈ 2 * 1.02 s ≈ 2.04 s
Scenario 2: Finding the Range
Using the same projectile as above, find the horizontal range (distance traveled).
- Solution:
- The horizontal distance is given by x = v₀ₓt. Using the time of flight calculated above:
- x = 17.32 m/s * 2.04 s ≈ 35.36 m
- The horizontal distance is given by x = v₀ₓt. Using the time of flight calculated above:
Scenario 3: Finding Maximum Height
What is the maximum height reached by the projectile?
- Solution:
- We can use y = v₀ᵧt - (1/2)gt², using the time to reach the highest point (1.02 s):
- y = 10(1.02) - (1/2)(9.8)(1.02)² ≈ 5.10 m
- We can use y = v₀ᵧt - (1/2)gt², using the time to reach the highest point (1.02 s):
Scenario 4: Projectile Launched Horizontally:
A ball is thrown horizontally from a cliff 50 meters high with an initial velocity of 15 m/s. How far from the base of the cliff will it land?
- Solution:
- First, find the time it takes to fall 50 meters using y = (1/2)gt² (since initial vertical velocity is 0).
- 50 = (1/2)(9.8)t² => t ≈ 3.19 s
- Then, find the horizontal distance using x = v₀ₓt:
- x = 15 m/s * 3.19 s ≈ 47.85 m
- First, find the time it takes to fall 50 meters using y = (1/2)gt² (since initial vertical velocity is 0).
Advanced Concepts and Considerations:
-
Air Resistance: In reality, air resistance significantly affects projectile motion. Air resistance opposes the motion of the projectile, leading to a shorter range and a less parabolic trajectory. Modeling air resistance requires more complex equations.
-
Varying Gravity: The acceleration due to gravity varies slightly with altitude and location. For most problems, the standard value of 9.8 m/s² is sufficient, but for high-precision calculations, this variation needs to be considered.
-
Multiple Projectiles: Problems can involve multiple projectiles interacting with each other or the environment. This adds another layer of complexity to the problem-solving process.
Conclusion:
This guide offers a thorough understanding of projectile motion, encompassing the fundamental principles, equations, problem-solving techniques, and advanced considerations. By mastering these concepts and practicing problem-solving, you'll be well-equipped to tackle various projectile motion challenges. Remember, consistent practice and a clear understanding of the underlying physics are key to success in this area. This in-depth exploration goes beyond a simple answer key, providing a robust foundation for future study in physics and related fields. Through detailed examples and step-by-step explanations, this resource aims to empower learners to confidently approach and solve complex projectile motion problems. The ability to accurately analyze and predict the trajectory of projectiles has far-reaching applications in various fields, highlighting the importance of a solid grasp of this fundamental kinematic concept.
Latest Posts
Latest Posts
-
Their Eyes Were Watching God Chapter 11
Mar 31, 2025
-
Which Of These Technological Advances Improved Flu
Mar 31, 2025
-
Plato The Republic Summary Book 2
Mar 31, 2025
-
Characters From The Importance Of Being Earnest
Mar 31, 2025
-
Othello Act 2 Scene 1 Summary
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about Unit 1 Kinematics 1.m Projectile Motion Answer Key . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
