Unit 7 Polygons And Quadrilaterals Homework 4 Rectangles Answer Key
Onlines
Apr 06, 2025 · 5 min read
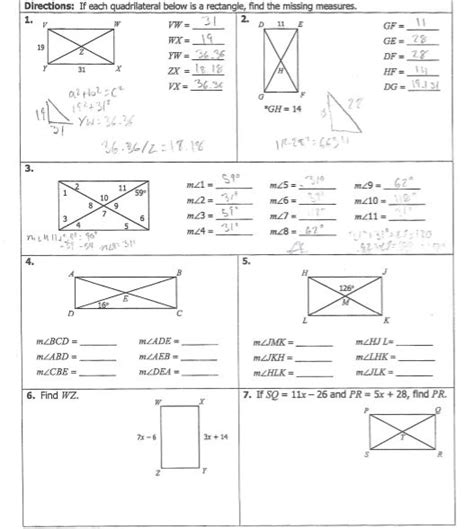
Table of Contents
Unit 7 Polygons and Quadrilaterals Homework 4 Rectangles: Answer Key and Comprehensive Guide
This comprehensive guide will delve into the concepts covered in Unit 7, Homework 4, focusing on rectangles. We'll explore the properties of rectangles, tackle common problem types, and provide solutions to help you solidify your understanding. This isn't just an answer key; it's a learning resource designed to build your geometric intuition and problem-solving skills.
Understanding Rectangles: A Foundation for Success
Before we jump into specific problems, let's review the defining characteristics of a rectangle. A rectangle is a quadrilateral (a four-sided polygon) with the following properties:
- Four right angles: Each interior angle measures exactly 90 degrees.
- Opposite sides are parallel and congruent: This means opposite sides have the same length and are parallel to each other.
- Diagonals bisect each other: The diagonals of a rectangle intersect at their midpoints, dividing each diagonal into two equal segments.
Understanding these properties is crucial for solving problems related to rectangles. Let's explore some common problem types.
Problem Type 1: Finding Missing Side Lengths
Many problems will involve finding the length of a missing side given other information. This often utilizes the Pythagorean theorem if diagonals are involved, or simply relies on the properties of opposite sides being equal.
Example: A rectangle has a length of 12 cm and a diagonal of 13 cm. Find the width of the rectangle.
Solution: We can use the Pythagorean theorem (a² + b² = c²) where 'a' and 'b' are the sides and 'c' is the diagonal.
- Let 'a' be the width (what we need to find).
- Let 'b' be the length (12 cm).
- Let 'c' be the diagonal (13 cm).
Therefore: a² + 12² = 13²
Solving for 'a':
a² + 144 = 169 a² = 25 a = 5 cm
The width of the rectangle is 5 cm.
Problem Type 2: Calculating Perimeter and Area
Calculating the perimeter and area of a rectangle are fundamental skills. Remember:
- Perimeter: The total distance around the outside of the rectangle. Formula: P = 2(length + width)
- Area: The space enclosed within the rectangle. Formula: A = length × width
Example: A rectangle has a length of 8 inches and a width of 5 inches. Calculate its perimeter and area.
Solution:
- Perimeter: P = 2(8 + 5) = 2(13) = 26 inches
- Area: A = 8 × 5 = 40 square inches
Problem Type 3: Working with Diagonals
Diagonals in rectangles create interesting geometric relationships. Remember that diagonals bisect each other (cut each other in half) and are congruent (have the same length).
Example: The diagonals of a rectangle intersect at point O. If one segment of a diagonal (from a vertex to O) measures 7 cm, what is the length of the entire diagonal?
Solution: Since the diagonals bisect each other, the entire diagonal is twice the length of the segment from a vertex to the intersection point. Therefore, the length of the entire diagonal is 2 × 7 cm = 14 cm.
Problem Type 4: Coordinate Geometry and Rectangles
Rectangles can be represented on a coordinate plane. Understanding how to find lengths using the distance formula and slopes is essential.
Example: A rectangle has vertices at A(2, 1), B(6, 1), C(6, 4), and D(2, 4). Find the length and width of the rectangle.
Solution: We can use the distance formula to find the distance between points: √[(x₂ - x₁)² + (y₂ - y₁)²]
- Length (AB or CD): √[(6 - 2)² + (1 - 1)²] = √(16 + 0) = 4 units
- Width (BC or AD): √[(6 - 6)² + (4 - 1)²] = √(0 + 9) = 3 units
Problem Type 5: Proofs and Geometric Reasoning
Some problems may require you to prove a specific property of a rectangle or use logical reasoning to deduce information.
Example: Prove that the diagonals of a rectangle are congruent.
Solution: This requires using the properties of a rectangle and possibly the Pythagorean theorem. A formal proof would involve stating given information, using postulates and theorems, and arriving at a conclusion. (This would typically be a multi-step proof and is best demonstrated visually with a diagram.)
Tackling Homework 4: A Step-by-Step Approach
Let's assume Homework 4 contains a series of problems similar to the examples above. A methodical approach is key:
-
Carefully read each problem: Understand what information is given and what you need to find. Draw a diagram if it helps visualize the problem.
-
Identify relevant properties: Determine which properties of rectangles are applicable to the specific problem (e.g., opposite sides are equal, diagonals bisect each other, etc.).
-
Choose appropriate formulas: Select the relevant formulas for perimeter, area, or the Pythagorean theorem as needed.
-
Solve the problem systematically: Show your work step-by-step, clearly indicating your calculations.
-
Check your answer: Does your answer make sense in the context of the problem? Are the units correct?
-
Review and learn from mistakes: If you get a problem wrong, review your steps and identify where you went wrong. This is crucial for improving your understanding.
Beyond the Answer Key: Developing Geometric Intuition
This guide is not just about providing answers; it's about fostering a deeper understanding of rectangles and related geometric concepts. To truly master this material, go beyond simply finding the answers. Consider the following:
-
Practice regularly: The more problems you solve, the more confident you'll become. Seek out additional practice problems if needed.
-
Visualize geometric concepts: Use diagrams, manipulatives (if available), or interactive geometry software to enhance your understanding of shapes and their properties.
-
Connect concepts: Look for connections between different geometric concepts and how they relate to rectangles.
-
Seek help when needed: If you are struggling with a particular concept, don't hesitate to seek help from your teacher, tutor, or classmates.
-
Explore advanced topics: Once you feel confident with the basics, explore more advanced topics related to quadrilaterals and polygons.
Conclusion: Mastering Rectangles and Beyond
This comprehensive guide has provided a detailed exploration of rectangles, including common problem types and a strategic approach to solving problems. Remember that true understanding comes from active engagement with the material, consistent practice, and a willingness to seek help when needed. By mastering the concepts covered in this unit, you'll build a strong foundation for more advanced geometric studies. Don't just aim for the correct answers; strive to understand why those answers are correct. This approach will lead to lasting comprehension and success in mathematics.
Latest Posts
Latest Posts
-
Introduction To Community Population Public And Global Health
Apr 07, 2025
-
Sariyah Sets Challenging Sales Targets For Her Team
Apr 07, 2025
-
Which Publication Covers Army Sop Requirements And Responsibilities
Apr 07, 2025
-
The Theme Of The Secret Garden
Apr 07, 2025
-
Ap Statistics Quiz 7 1 A Answers
Apr 07, 2025
Related Post
Thank you for visiting our website which covers about Unit 7 Polygons And Quadrilaterals Homework 4 Rectangles Answer Key . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
