Which Of The Following Is The Empirical Formula For C12h18o6
Onlines
Mar 29, 2025 · 5 min read
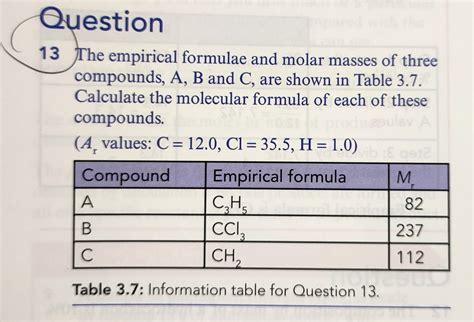
Table of Contents
Determining the Empirical Formula for C₁₂H₁₈O₆
The question "Which of the following is the empirical formula for C₁₂H₁₈O₆?" presupposes a multiple-choice answer set which isn't provided. However, we can definitively determine the empirical formula for C₁₂H₁₈O₆ and explore the concept of empirical formulas in greater detail. This will allow you to answer any multiple-choice question related to this specific chemical formula and provide a solid understanding of the topic.
Understanding Empirical Formulas
An empirical formula represents the simplest whole-number ratio of atoms of each element present in a compound. It doesn't necessarily reflect the actual number of atoms in a molecule (the molecular formula), but rather the smallest ratio possible. Think of it as the most reduced form of the chemical formula.
For example, glucose has a molecular formula of C₆H₁₂O₆. To find the empirical formula, we look for the greatest common divisor (GCD) of the subscripts. In this case, the GCD of 6, 12, and 6 is 6. Dividing each subscript by 6, we get the empirical formula: CH₂O. This means that for every carbon atom, there are two hydrogen atoms and one oxygen atom, irrespective of the actual number of atoms present in a single glucose molecule.
Finding the Empirical Formula for C₁₂H₁₈O₆
Let's apply this to C₁₂H₁₈O₆. We need to find the GCD of the subscripts 12, 18, and 6.
- Factors of 12: 1, 2, 3, 4, 6, 12
- Factors of 18: 1, 2, 3, 6, 9, 18
- Factors of 6: 1, 2, 3, 6
The greatest common factor among these three numbers is 6. Therefore, we divide each subscript in the molecular formula C₁₂H₁₈O₆ by 6:
- C₁₂/₆ = C₂
- H₁₈/₆ = H₃
- O₆/₆ = O₁
Therefore, the empirical formula for C₁₂H₁₈O₆ is C₂H₃O.
Distinguishing Molecular and Empirical Formulas
It's crucial to understand the difference between molecular and empirical formulas:
-
Molecular Formula: Shows the actual number of atoms of each element in a molecule. This is the true composition of a single molecule. Example: C₆H₁₂O₆ (glucose)
-
Empirical Formula: Shows the simplest whole-number ratio of atoms in a compound. It's the most reduced form of the molecular formula. Example: CH₂O (glucose)
Many compounds share the same empirical formula but have different molecular formulas. This is because the molecular formula is a multiple of the empirical formula. For instance, formaldehyde (CH₂O), acetic acid (C₂H₄O₂), and glucose (C₆H₁₂O₆) all have the same empirical formula (CH₂O), but their molecular formulas and properties differ significantly.
Determining Empirical Formulas from Experimental Data
Often, you won't be given the molecular formula directly. Instead, you'll need to determine the empirical formula from experimental data, typically obtained through combustion analysis or other quantitative methods. This involves:
-
Determining the mass of each element present: This might involve techniques like combustion analysis, where a sample is burned in oxygen, and the masses of CO₂, H₂O, and other products are measured. From this, the mass of carbon, hydrogen, and oxygen in the original sample can be calculated.
-
Converting mass to moles: Using the molar mass of each element, convert the mass of each element to moles.
-
Finding the mole ratio: Divide the number of moles of each element by the smallest number of moles obtained. This gives the simplest whole-number ratio of atoms, which is the empirical formula.
-
Adjusting to whole numbers: If the mole ratios are not whole numbers, you may need to multiply them by a small integer to obtain whole numbers.
Example: Let's say combustion analysis reveals a compound contains 40.0% carbon, 6.7% hydrogen, and 53.3% oxygen by mass.
-
Assume a 100g sample: This simplifies calculations. We have 40.0g C, 6.7g H, and 53.3g O.
-
Convert to moles:
- Moles of C = 40.0g / 12.01 g/mol ≈ 3.33 mol
- Moles of H = 6.7g / 1.01 g/mol ≈ 6.63 mol
- Moles of O = 53.3g / 16.00 g/mol ≈ 3.33 mol
-
Find mole ratio: Divide by the smallest number of moles (3.33):
- C: 3.33 mol / 3.33 mol = 1
- H: 6.63 mol / 3.33 mol ≈ 2
- O: 3.33 mol / 3.33 mol = 1
-
Empirical Formula: The empirical formula is CH₂O.
Applications of Empirical Formulas
Empirical formulas are crucial in various chemical contexts:
-
Identifying unknown compounds: By determining the empirical formula, chemists can narrow down the possibilities for an unknown compound's identity.
-
Determining molecular formulas: Combined with the molar mass of the compound, the empirical formula can be used to calculate the molecular formula.
-
Stoichiometric calculations: Empirical formulas are essential for performing stoichiometric calculations, which involve relating the amounts of reactants and products in a chemical reaction.
-
Understanding chemical composition: Empirical formulas provide fundamental insights into the relative proportions of elements within a compound.
Further Considerations and Advanced Topics
While this discussion covers the basics of empirical formulas and their determination, more advanced aspects exist. These include:
-
Dealing with hydrates: Hydrates are compounds that include water molecules in their crystal structure. These water molecules must be considered when determining the empirical formula.
-
Using more complex analytical techniques: Advanced techniques such as mass spectrometry, nuclear magnetic resonance (NMR) spectroscopy, and X-ray crystallography provide more detailed information about the molecular structure and composition, often bypassing the need for simple combustion analysis.
Understanding empirical formulas is a fundamental aspect of chemistry. Mastering this concept allows for better interpretation of experimental data, deeper understanding of chemical composition, and proficiency in solving various chemistry problems, particularly those involving stoichiometry and the determination of unknown compounds. Remember, the key is to find the simplest whole-number ratio of atoms—the essence of the empirical formula.
Latest Posts
Latest Posts
-
Which Of The Following Is An Example Of Green Computing
Mar 31, 2025
-
Summary For Chapter 11 To Kill A Mockingbird
Mar 31, 2025
-
Which Drive Is Displayed First In The Command Window
Mar 31, 2025
-
Which Of The Following Diagrams Involves A Virtual Image
Mar 31, 2025
-
Which Of The Following Statements About Gerrymandering Is True
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about Which Of The Following Is The Empirical Formula For C12h18o6 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
