8 3 Practice Special Right Triangles
Onlines
Mar 28, 2025 · 6 min read
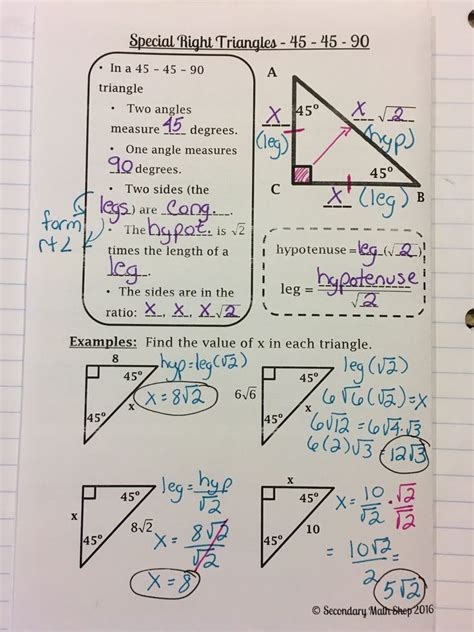
Table of Contents
Mastering 8.3 Practice: Special Right Triangles
Special right triangles—the 30-60-90 and 45-45-90 triangles—are fundamental geometric shapes with unique properties that simplify many mathematical problems. Understanding their ratios and applying them effectively is crucial for success in geometry and trigonometry. This comprehensive guide will delve into the intricacies of these triangles, providing you with a solid foundation to tackle even the most challenging problems involving 8.3 practice exercises. We'll cover the fundamental ratios, explore various problem-solving techniques, and work through numerous examples to solidify your understanding.
Understanding the 30-60-90 Triangle
The 30-60-90 triangle, also known as a half-equilateral triangle, is formed by bisecting an equilateral triangle. This results in a triangle with angles measuring 30°, 60°, and 90°. The key to solving problems involving 30-60-90 triangles lies in understanding its side ratios.
The Ratio: The sides of a 30-60-90 triangle are always in the ratio of 1 : √3 : 2. Specifically:
- Shortest side (opposite the 30° angle): 1x
- Medium side (opposite the 60° angle): √3x
- Hypotenuse (opposite the 90° angle): 2x
Where 'x' is a scaling factor that can be any positive number.
Example 1: Finding Missing Sides
Let's say we have a 30-60-90 triangle where the shortest side (opposite the 30° angle) measures 5 cm. Using the ratio, we can find the lengths of the other sides:
- Shortest side = 1x = 5 cm Therefore, x = 5 cm
- Medium side = √3x = √3 * 5 cm = 5√3 cm
- Hypotenuse = 2x = 2 * 5 cm = 10 cm
Example 2: Finding the Angle
If you are given the length of two sides, you can use the ratio to determine if it’s a 30-60-90 triangle. Let's say we have a right-angled triangle with sides of 7 cm and 7√3 cm. Since the ratio of the sides is 7 : 7√3, which simplifies to 1 : √3, we know that this is a 30-60-90 triangle. The angle opposite the side of 7 cm is 30°, and the angle opposite the side of 7√3 cm is 60°.
Example 3: Area Calculation
The area of a triangle is given by the formula: Area = (1/2) * base * height. In a 30-60-90 triangle, we can choose either the side opposite the 30° angle or the side opposite the 60° angle as the base, with the other side being the height.
Consider a 30-60-90 triangle with the shortest side (opposite 30°) as 6. The medium side (opposite 60°) is then 6√3 and the hypotenuse is 12.
Area = (1/2) * 6 * 6√3 = 18√3 square units.
Understanding the 45-45-90 Triangle
The 45-45-90 triangle, also known as an isosceles right triangle, has two angles of 45° and one right angle (90°). Because it's isosceles, two of its sides are equal in length.
The Ratio: The side ratios of a 45-45-90 triangle are 1 : 1 : √2.
- Legs (opposite the 45° angles): 1x
- Hypotenuse (opposite the 90° angle): √2x
Example 4: Finding Missing Sides
If one leg of a 45-45-90 triangle measures 8 cm, then the other leg also measures 8 cm. The hypotenuse would be 8√2 cm.
Example 5: Finding the Angle
If you're given a right-angled triangle with two equal sides, you immediately know it's a 45-45-90 triangle, and both angles opposite the equal sides will measure 45°.
Example 6: Applications in Geometry
45-45-90 triangles frequently appear in geometric problems involving squares (when a diagonal is drawn) and other symmetrical figures. Understanding their properties is key to finding the lengths of diagonals, areas, and other geometric properties.
Advanced Problem Solving Techniques
Many 8.3 practice problems will involve a combination of special right triangles and other geometric principles. Here are some advanced techniques:
1. Breaking Down Complex Shapes: Complex shapes can often be broken down into simpler shapes, including special right triangles. By identifying these triangles, you can solve for unknown side lengths and angles.
2. Using Trigonometric Functions: While not strictly necessary for solving problems involving only the sides of special right triangles, trigonometric functions (sine, cosine, tangent) can be helpful when dealing with angles and unknown sides in more complex scenarios involving these triangles within a larger geometric shape.
3. Applying the Pythagorean Theorem: The Pythagorean theorem (a² + b² = c²) is always valid in right-angled triangles, including special right triangles. It can be used to verify your solutions or to solve for unknown sides when other methods are insufficient.
4. Coordinate Geometry: Special right triangles frequently appear in coordinate geometry problems. Understanding how to locate vertices of triangles on a Cartesian plane and apply distance formulas alongside the properties of special right triangles is critical for success in these types of problems.
Practice Problems and Solutions
Let's work through a few practice problems to solidify your understanding:
Problem 1: A 30-60-90 triangle has a hypotenuse of length 12 cm. Find the lengths of the other two sides.
Solution: Since the hypotenuse is 2x = 12 cm, x = 6 cm. The shortest side is 1x = 6 cm, and the medium side is √3x = 6√3 cm.
Problem 2: An isosceles right triangle has a leg of length 5 cm. Find the length of the hypotenuse.
Solution: The hypotenuse is √2 times the length of a leg, so it’s 5√2 cm.
Problem 3: A square has a side length of 10 cm. Find the length of its diagonal.
Solution: The diagonal of a square forms two 45-45-90 triangles. The diagonal is √2 times the side length, so it’s 10√2 cm.
Problem 4: A right-angled triangle has sides of length 4 and 4√3. Determine the type of triangle and find the length of the hypotenuse.
Solution: The ratio of the sides is 4 : 4√3, which simplifies to 1 : √3. This is a 30-60-90 triangle. The hypotenuse is 2 times the shortest side, which is 2 * 4 = 8.
Problem 5: A rectangle has a length of 12 and a diagonal of 13. Find the width.
Solution: This problem creates a right-angled triangle with hypotenuse 13 and one leg 12. Using the Pythagorean theorem: width² + 12² = 13². Therefore, width² = 169 - 144 = 25, and the width = 5.
Conclusion
Mastering special right triangles is essential for success in various mathematical applications. By thoroughly understanding their side ratios, applying problem-solving techniques, and practicing consistently, you can confidently tackle a wide range of problems. Remember to break down complex shapes into simpler ones and utilize the Pythagorean theorem when necessary. Consistent practice with diverse problems is the key to building a strong understanding of 8.3 practice exercises on special right triangles. Through dedicated study and application of the concepts covered in this guide, you can achieve mastery and excel in your geometric endeavors. Remember to review and practice regularly to reinforce your learning and build confidence in solving problems involving these essential geometric shapes.
Latest Posts
Latest Posts
-
What Pattern Did You See Repeated Every Year
Mar 31, 2025
-
Which Of The Following Nutrients Do Infants Require Extra Servings
Mar 31, 2025
-
Wordly Wise 3000 Book 12 Answer Key
Mar 31, 2025
-
Classify Each Molecule As An Aldehyde Ketone Or Neither
Mar 31, 2025
-
Ap Chem Unit 1 Progress Check Mcq
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about 8 3 Practice Special Right Triangles . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
