Experiment 4 Density Of The Mass Set
Onlines
Apr 05, 2025 · 5 min read
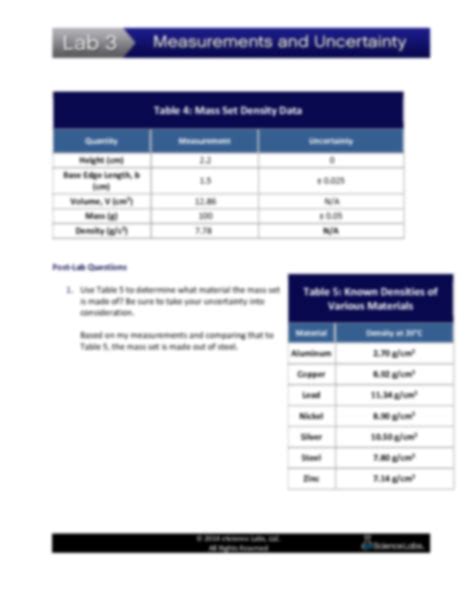
Table of Contents
Experiment 4: Density of the Mass Set – A Deep Dive into Measurement and Analysis
This article provides a comprehensive guide to Experiment 4, focusing on determining the density of a mass set. We'll delve into the theoretical background, step-by-step procedure, data analysis techniques, potential sources of error, and best practices for enhancing experimental accuracy. This detailed exploration will equip you with the knowledge to successfully conduct and interpret this crucial physics experiment.
Understanding Density: The Foundation of the Experiment
Density is a fundamental physical property of matter, defined as the mass per unit volume. It's a crucial concept across various scientific disciplines, from material science to fluid mechanics. The formula for density is:
Density (ρ) = Mass (m) / Volume (V)
Where:
- ρ (rho) represents density, typically measured in kg/m³ or g/cm³.
- m represents mass, typically measured in kilograms (kg) or grams (g).
- V represents volume, typically measured in cubic meters (m³) or cubic centimeters (cm³).
Understanding this formula is the cornerstone of successfully conducting and interpreting Experiment 4. The experiment aims to determine the density of various masses within a mass set, allowing for the verification of material properties and the exploration of measurement techniques.
The Experimental Setup: Tools and Materials
To perform Experiment 4 accurately, you will need the following materials and equipment:
- A Mass Set: This is the core of the experiment, providing the various masses whose densities you'll determine. The mass set should ideally contain a range of masses for robust data analysis.
- A Vernier Caliper: This precision instrument is crucial for accurately measuring the dimensions of the masses. Its ability to measure to the nearest 0.01mm or 0.001 inch is vital for accurate volume calculation.
- A Triple-Beam Balance or Electronic Balance: This is used for precisely measuring the mass of each object in the mass set. Ensure your chosen balance is calibrated correctly for optimal accuracy.
- A Ruler: While less precise than a vernier caliper, a ruler can be used as a supplementary tool for measuring the dimensions, especially for irregularly shaped masses.
- Graph Paper or Spreadsheet Software: This is essential for recording your data and creating graphs to visualize your results. Spreadsheet software like Excel or Google Sheets offers powerful tools for data analysis.
- Calculator: Necessary for performing calculations related to volume and density.
- Water (Optional): If the masses are irregularly shaped, using water displacement is a common technique for determining volume, requiring a graduated cylinder.
Procedure: A Step-by-Step Guide
The following steps outline a systematic approach to conducting Experiment 4:
Step 1: Prepare the Equipment
Ensure all measuring instruments (balance, vernier caliper, ruler) are properly calibrated and clean. Check for any damage or malfunctions that could compromise the accuracy of your measurements.
Step 2: Measure the Mass
Carefully measure the mass of each object in the mass set using the balance. Record the mass (m) for each object in a well-organized data table. Repeat the mass measurement multiple times for each object to minimize the impact of random errors. Calculate the average mass for each object.
Step 3: Measure the Dimensions
This step is crucial and depends on the shape of the masses in your set.
-
Regular Shapes (e.g., cubes, cylinders): Use the vernier caliper to accurately measure the length, width, and height (for cubes) or the diameter and height (for cylinders) of each object. Record these dimensions in your data table.
-
Irregular Shapes: Measuring irregular shapes requires a different approach. One common method is water displacement:
- Fill a graduated cylinder partially with water, recording the initial volume.
- Carefully submerge the object completely in the water, ensuring no air bubbles are trapped.
- Record the new water level.
- The difference between the two water levels represents the volume of the object.
Step 4: Calculate the Volume
Using the measured dimensions, calculate the volume (V) of each object:
- Cubes: V = length x width x height
- Cylinders: V = πr²h (where r is the radius and h is the height)
- Irregular Shapes: V = difference in water levels (obtained through water displacement)
Step 5: Calculate the Density
For each object, use the formula ρ = m/V to calculate the density. Record the calculated density in your data table.
Step 6: Data Analysis and Graphing
Organize your data into a clear and concise table. Include columns for the mass, dimensions (length, width, height, diameter, etc.), volume, and density for each object. Use spreadsheet software to create a graph showing the relationship between mass and volume (mass on the y-axis, volume on the x-axis). The slope of this graph should represent the average density of the mass set.
Step 7: Error Analysis
Analyze the sources of error in your experiment. Consider potential errors in measurement (e.g., parallax error when using the vernier caliper, uncertainty in the balance reading), variations in material composition, and inconsistencies in shape. Calculate the percentage error for each density measurement to quantify the accuracy of your results.
Advanced Techniques and Considerations
- Uncertainty Analysis: Include uncertainty estimations for all measurements (mass, dimensions). Propagate these uncertainties through your calculations to determine the uncertainty in your density calculations.
- Statistical Analysis: For multiple measurements of mass and dimensions, calculate the average and standard deviation to represent the central tendency and dispersion of your data.
- Material Identification: Compare the calculated density to known densities of common materials to identify the potential composition of the mass set's components.
- Density of Irregular Objects: Water displacement, while effective, can be prone to errors due to air bubbles. Alternative techniques like using a pycnometer (a specialized instrument for precise volume measurement) can significantly enhance accuracy.
- Temperature Considerations: Density is temperature-dependent; accounting for temperature variations can improve the accuracy of your results.
Conclusion: Interpreting Results and Drawing Meaningful Conclusions
Experiment 4 offers valuable insights into the concept of density and the importance of accurate measurement techniques. By meticulously following the procedure, analyzing data effectively, and understanding potential error sources, you can develop a comprehensive understanding of this fundamental physical property. The ability to precisely measure and analyze the density of materials is crucial across diverse scientific and engineering fields. The skills honed during this experiment form the basis for more complex experimental work in the future. Always remember to clearly communicate your findings, including your methodology, data, analysis, and conclusions, in a well-structured report. This approach demonstrates scientific rigor and enables others to understand and replicate your work. The ability to clearly and concisely communicate scientific results is as important as the scientific method itself.
Latest Posts
Latest Posts
-
The Main Categories Of Supports For Individuals Include
Apr 05, 2025
-
Power And Influence A Managerial Thrill Ride
Apr 05, 2025
-
8 5 Volumes By Cross Sections Homework
Apr 05, 2025
-
Is Most Well Known For Proposing His Hierarchy Of Needs
Apr 05, 2025
-
Theme For The Raven By Edgar Allan Poe
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about Experiment 4 Density Of The Mass Set . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
