Solving Rational Equations Quiz Part 1
Onlines
Mar 31, 2025 · 4 min read
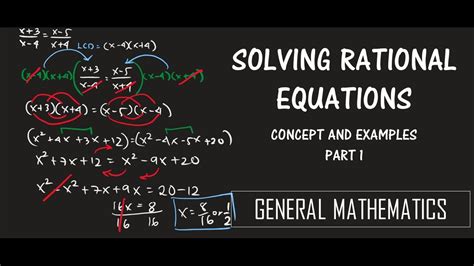
Table of Contents
Solving Rational Equations Quiz: Part 1 - Mastering the Fundamentals
Rational equations, those equations containing fractions with variables in the denominator, can seem daunting at first. However, with a systematic approach and a solid understanding of fundamental algebraic principles, conquering these equations becomes significantly easier. This comprehensive guide serves as your ultimate resource for mastering rational equations, complete with a quiz to test your understanding. Let's dive in!
Understanding Rational Equations
A rational equation is an equation where the variable appears in the denominator of at least one fraction. For example, x/(x-2) + 1 = 3/(x-2) is a rational equation. Solving these equations involves manipulating the equation to eliminate the fractions and then solving the resulting polynomial equation.
Key Concepts:
- Finding the Least Common Denominator (LCD): This is crucial for eliminating fractions. The LCD is the smallest expression that is a multiple of all the denominators in the equation.
- Extraneous Solutions: These are solutions that appear to satisfy the equation after solving, but actually make the denominator zero in the original equation. They are invalid solutions and must be excluded. Always check your solutions by plugging them back into the original equation.
- Factoring: Often, factoring the denominators is necessary to find the LCD and to simplify the equation.
Step-by-Step Solution Process
Solving rational equations involves a systematic approach:
- Identify the LCD: Find the least common denominator of all the fractions in the equation.
- Multiply by the LCD: Multiply both sides of the equation by the LCD. This will eliminate the fractions.
- Simplify and Solve: Simplify the resulting equation and solve it using appropriate algebraic techniques (like factoring, the quadratic formula, etc.).
- Check for Extraneous Solutions: Substitute each solution back into the original equation to ensure it doesn't make any denominator equal to zero. Discard any extraneous solutions.
Example Problems
Let's work through a few examples to solidify our understanding:
Example 1: Simple Rational Equation
Solve: 1/x + 2/3x = 5/6
- LCD: The LCD of x and 3x is 3x.
- Multiply by LCD: Multiply both sides by 3x:
3x(1/x + 2/3x) = 3x(5/6) - Simplify:
3 + 2 = 5x/2which simplifies to5 = 5x/2 - Solve: Multiply both sides by 2/5:
x = 2 - Check: Substitute x = 2 into the original equation:
1/2 + 2/(3*2) = 1/2 + 1/3 = 5/6. The solution is valid.
Therefore, x = 2
Example 2: Equation with Factoring
Solve: (x+1)/(x-2) - 2/(x+2) = (x²+6)/(x²-4)
- Factor the Denominators: Note that x²-4 = (x-2)(x+2). The denominators are (x-2), (x+2) and (x-2)(x+2).
- LCD: The LCD is (x-2)(x+2).
- Multiply by LCD: Multiply both sides by (x-2)(x+2):
(x+1)(x+2) - 2(x-2) = x²+6 - Simplify and Solve: Expanding and simplifying the equation:
x²+3x+2 - 2x+4 = x²+6which simplifies tox²+x+6 = x²+6. Subtracting x² and 6 from both sides givesx = 0. - Check: Substitute x = 0 into the original equation:
(0+1)/(0-2) - 2/(0+2) = -1/2 - 1 = -3/2. The right side is (0+6)/(0-4) = -3/2. The solution is valid.
Therefore, x = 0
Example 3: Equation with an Extraneous Solution
Solve: 2/x + 1/(x-1) = (2x-1)/(x(x-1))
- LCD: The LCD is x(x-1).
- Multiply by LCD:
2(x-1) + x = 2x-1 - Simplify and Solve:
2x - 2 + x = 2x - 1which simplifies tox = 1. - Check: Substitute x = 1 into the original equation: We get a division by zero, making x = 1 an extraneous solution.
Therefore, there is no solution to this equation.
Quiz Time!
Now, let's test your understanding with a quiz. Solve the following rational equations, remembering to check for extraneous solutions:
Question 1:
3/x - 1/(x+1) = 2/(x(x+1))
Question 2:
(x+2)/(x-3) + (x-2)/(x+3) = (2x² + 16)/(x²-9)
Question 3:
1/(x-2) + 1/(x+2) = 4/(x²-4)
Question 4:
2/(x+1) - 1/(x-1) = 1/(x²-1)
Question 5:
5/(x-3) + 3/(x+3) = 24/(x²-9)
Solutions (Hidden for Self-Assessment)
<details> <summary>Click to reveal solutions</summary>
Solution 1: x = 2 Solution 2: x = 0 Solution 3: No solution (extraneous solution) Solution 4: x = 2 Solution 5: x = 1
</details>
Advanced Techniques
While the above examples cover the basics, more complex rational equations may require additional techniques:
- Partial Fraction Decomposition: This technique is used to break down complex rational expressions into simpler fractions, making them easier to solve.
- Substitution: Sometimes, substituting a variable can simplify the equation significantly.
- Graphing: Graphing the equation can help visualize the solutions and identify potential extraneous solutions.
Conclusion
Solving rational equations involves a systematic approach that combines several algebraic techniques. Remember to always find the LCD, eliminate the fractions, solve the resulting equation, and check for extraneous solutions. This comprehensive guide and the practice quiz should equip you with the skills to confidently tackle a wide range of rational equation problems. Remember to practice regularly to build your fluency and understanding. Good luck!
Latest Posts
Latest Posts
-
Which Of These Statements About The Elderly Is True
Apr 01, 2025
-
The Bible Itself Has Never Really Faced Extreme Persecution
Apr 01, 2025
-
The Team Functions Smoothly When All Team Members
Apr 01, 2025
-
To Which Patient Might The Nurse Apply A Physical Restraint
Apr 01, 2025
-
Summary Of Chapter 5 Into The Wild
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Solving Rational Equations Quiz Part 1 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
