Worksheet 7.4 Inverse Functions Answer Key
Onlines
Mar 30, 2025 · 5 min read
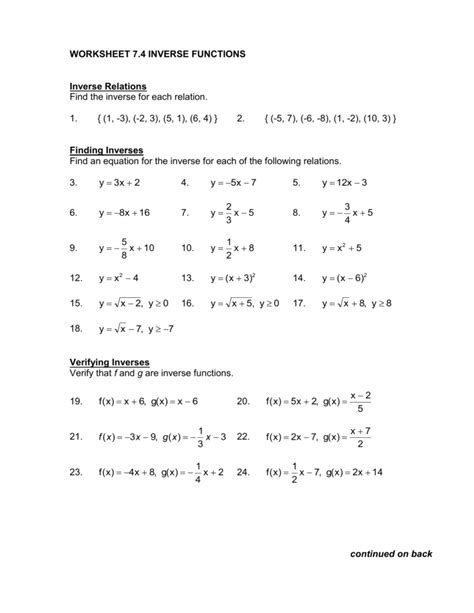
Table of Contents
- Worksheet 7.4 Inverse Functions Answer Key
- Table of Contents
- Worksheet 7.4 Inverse Functions: A Comprehensive Guide with Answers
- Understanding Inverse Functions
- Finding the Inverse of a Function
- Verifying Inverse Functions
- Common Mistakes to Avoid
- Addressing Specific Worksheet Challenges (General Approach)
- Strategies for Success
- Latest Posts
- Latest Posts
- Related Post
Worksheet 7.4 Inverse Functions: A Comprehensive Guide with Answers
Finding the answer key for Worksheet 7.4 on inverse functions can be tricky. Many resources are scattered across the internet, and the specific worksheet content varies depending on the textbook or curriculum used. This comprehensive guide aims to provide a thorough understanding of inverse functions, equipping you with the knowledge to solve problems similar to those found on Worksheet 7.4, regardless of its specific contents. We will cover key concepts, provide examples, and offer a general approach to solving inverse function problems. While we can't provide the exact answers to your specific worksheet, this guide will empower you to find them independently.
Understanding Inverse Functions
An inverse function essentially "undoes" what the original function does. If function f maps x to y, then its inverse function, denoted as f<sup>-1</sup>(x), maps y back to x. This relationship is only possible if the original function is one-to-one, meaning each input has a unique output, and vice-versa. Graphically, a one-to-one function passes both the vertical and horizontal line tests.
Key Characteristics of Inverse Functions:
- Domain and Range Swap: The domain of f(x) becomes the range of f<sup>-1</sup>(x), and vice-versa.
- Composition Property: f(f<sup>-1</sup>(x)) = x and f<sup>-1</sup>(f(x)) = x. This means applying the function and its inverse consecutively results in the original input.
- Reflection Symmetry: The graphs of f(x) and f<sup>-1</sup>(x) are reflections of each other across the line y = x.
Finding the Inverse of a Function
The process of finding the inverse of a function involves several steps:
-
Replace f(x) with y: This simplifies the notation.
-
Swap x and y: This reflects the domain and range swap characteristic.
-
Solve for y: Algebraically manipulate the equation to isolate y.
-
Replace y with f<sup>-1</sup>(x): This denotes the inverse function.
Example 1: Finding the Inverse of a Linear Function
Let's find the inverse of the function f(x) = 2x + 3.
-
y = 2x + 3
-
x = 2y + 3
-
x - 3 = 2y y = (x - 3)/2
-
f<sup>-1</sup>(x) = (x - 3)/2
Example 2: Finding the Inverse of a Quadratic Function (Restricted Domain)
Quadratic functions are not one-to-one over their entire domain. To find their inverse, we must restrict the domain. Consider f(x) = x², with the domain restricted to x ≥ 0.
-
y = x²
-
x = y²
-
y = √x (We take the positive square root because of the restricted domain)
-
f<sup>-1</sup>(x) = √x
Example 3: Finding the Inverse of a More Complex Function
Let's consider f(x) = (3x - 1) / (x + 2).
-
y = (3x - 1) / (x + 2)
-
x = (3y - 1) / (y + 2)
-
x(y + 2) = 3y - 1 xy + 2x = 3y - 1 xy - 3y = -2x - 1 y(x - 3) = -2x - 1 y = (-2x - 1) / (x - 3)
-
f<sup>-1</sup>(x) = (-2x - 1) / (x - 3)
Verifying Inverse Functions
After finding the inverse, it's crucial to verify the result using the composition property: f(f<sup>-1</sup>(x)) = x and f<sup>-1</sup>(f(x)) = x.
Example: Verifying the Inverse from Example 1
We found f<sup>-1</sup>(x) = (x - 3)/2 for f(x) = 2x + 3. Let's verify:
f(f<sup>-1</sup>(x)) = 2((x - 3)/2) + 3 = x - 3 + 3 = x*
f<sup>-1</sup>(f(x)) = ((2x + 3) - 3)/2 = (2x)/2 = x
The composition property holds true, confirming our inverse function is correct.
Common Mistakes to Avoid
- Forgetting to swap x and y: This is a fundamental step in finding the inverse.
- Incorrect algebraic manipulation: Pay close attention to solving for y accurately.
- Ignoring domain restrictions: This is especially important for functions like quadratic and radical functions.
- Not verifying the inverse: Verification ensures the accuracy of your solution.
Addressing Specific Worksheet Challenges (General Approach)
While we lack the exact questions from Worksheet 7.4, let's address potential problem types:
1. Graphing Inverse Functions: If your worksheet includes graphing, remember that the graphs of f(x) and f<sup>-1</sup>(x) are reflections across the line y = x. You can plot points from the original function and then reflect those points across y = x to obtain the graph of the inverse.
2. Finding the Inverse of Piecewise Functions: Piecewise functions are defined by different expressions over different intervals. To find the inverse, you need to find the inverse for each piece separately, and then adjust the domains accordingly.
3. Working with Trigonometric Functions: The inverse trigonometric functions (arcsin, arccos, arctan, etc.) have restricted domains and ranges. Ensure you understand these restrictions when working with them.
4. Determining if a Function Has an Inverse: Remember, a function must be one-to-one to have an inverse. Check using the horizontal line test. If a horizontal line intersects the graph more than once, the function doesn't have an inverse over its entire domain.
Strategies for Success
- Practice: The more problems you solve, the better you'll understand the concepts and techniques.
- Understand the concepts: Don't just memorize steps; understand the underlying principles of inverse functions.
- Seek help when needed: Don't hesitate to ask your teacher, classmates, or tutor for assistance if you're struggling.
- Use online resources: While there isn't a single answer key for Worksheet 7.4, many online resources can provide examples and explanations to help you understand the concepts.
This comprehensive guide provides a robust foundation in inverse functions, empowering you to tackle problems similar to those found in Worksheet 7.4. Remember to focus on understanding the concepts, practice diligently, and verify your solutions. With consistent effort, you'll master the art of working with inverse functions.
Latest Posts
Latest Posts
-
Should Your Captors Provide An Opportunity
Apr 02, 2025
-
The Term Meritocracy Is Defined By The Text As
Apr 02, 2025
-
You Have To Cross A Broad River
Apr 02, 2025
-
Label The Transmission Electron Micrograph Of The Nucleus
Apr 02, 2025
-
Your Clients Target Demographic Is In Boston
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Worksheet 7.4 Inverse Functions Answer Key . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
